[go: up one dir, main page]
Search a number
katadromes
A number is a katadrome in a given base 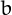 $b$  (often 10 or 16) if its digits are in strictly decreasing order in that base.

For example, 43210, 76521 and 9630 are all katadromes in base 10.

If we allow the digits of a katadrome to be non-strictly decreasing (i.e., nonincreasing, like in 43310 or 2222, we obtain nialpdromes.

Similarly, the numbers whose digits are nondecreasing and strictly increasing are called plaindromes and metadromes, respectively.

The total number katadromes in base  $b$  is equal to  $2^b-1$, hence in base 10 there are  $2^{10}-1 = 1023$  katadromes, from 0 to 9876543210.

 $p_{8510}=87641$  is the largest katadromic prime whose index is a katadromic too.

The first katadromes (in base 10) are 0, 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 20, 21, 30, 31, 32, 40, 41, 42, 43, 50 more terms

Pictorial representation of remainders (mod 2, 3, ...,11) frequency. For a table of values and more details click here
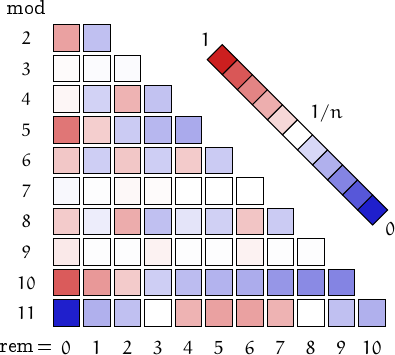
remainders of katadromes

A graph displaying how many katadromes are multiples of the primes p from 2 to 71. In black the ideal line 1/p.
divisibility of katadromes
Useful links Mathworld, Katadrome
OEIS, Sequence A009995 (base 10)
OEIS, Sequence A023797 (base 16)
Katadromes can also be... (you may click on names or numbers and on + to get more values)

a-pointer 631 8543 ABA 32 50 64 + 98 aban 10 20 21 + 987 abundant 20 30 40 + 9876540 Achilles 72 432 864 972 admirable 20 30 40 + 76542 alternating 10 21 30 + 987654321 amenable 20 21 32 + 987654321 apocalyptic 540 541 610 + 9876 arithmetic 20 21 30 + 9876543 automorphic 76 balanced p. 53 653 9871 96431 Bell 52 betrothed 75 binomial 10 20 21 + 986310 brilliant 10 21 731 + 9876431 c.decagonal 31 61 c.heptagonal 43 71 841 953 c.nonagonal 10 91 820 + 86320 c.octagonal 81 841 961 c.pentagonal 31 51 76 + 987531 c.square 41 61 85 + 8321 c.triangular 10 31 64 + 7543210 cake 42 64 93 Catalan 42 Chen 31 41 53 + 9875321 congruent 20 21 30 + 9876543 constructible 10 20 30 + 960 cube 64 Cullen 65 Cunningham 10 31 50 + 9410 Curzon 21 30 41 + 98765421 cyclic 31 41 43 + 9876541 D-number 21 51 63 + 987621 d-powerful 43 63 5320 + 9876532 de Polignac 6521 7431 8621 + 98654321 decagonal 10 52 85 + 9850 deceptive 91 6541 deficient 10 21 31 + 9876543 dig.balanced 10 21 41 + 9876540 droll 72 Duffinian 21 32 50 + 9876541 eban 30 32 40 + 64 economical 10 21 31 + 9875321 emirp 31 71 73 + 9875321 emirpimes 51 62 85 + 98754321 equidigital 10 21 31 + 9875321 eRAP 20 98 esthetic 10 21 32 + 9876543210 evil 10 20 30 + 987654320 factorial 720 fibodiv 61 75 87321 Fibonacci 21 610 987 Friedman 765432 976521 976532 976542 gapful 3210 4320 6420 + 9876543210 Giuga 30 good prime 41 53 71 + 8521 happy 10 31 32 + 9876543 Harshad 10 20 21 + 9876543210 heptagonal 81 540 874 970 hex 61 91 631 + 876421 hexagonal 91 630 861 + 86320 highly composite 60 720 840 hoax 84 85 94 + 9876210 Hogben 21 31 43 + 87321 house 32 652 hungry 74 hyperperfect 21 iban 10 20 21 + 743210 idoneal 10 21 30 + 840 impolite 32 64 inconsummate 62 63 65 + 987650 interprime 21 30 42 + 98765310 Jacobsthal 21 43 85 Jordan-Polya 32 64 72 + 8640 junction 210 410 420 + 9876543 Lehmer 51 85 91 763 Leyland 32 54 320 lonely 53 Lucas 76 521 843 lucky 21 31 43 + 9876541 Lynch-Bell 432 864 6432 + 9864 m-pointer 61 magic 65 870 magnanimous 20 21 30 + 9752 modest 654 721 763 + 96432 Moran 21 42 63 + 87543210 Motzkin 21 51 nialpdrome 10 20 21 + 9876543210 nonagonal 75 651 750 nude 432 864 6432 + 9864 O'Halloran 20 60 84 420 oban 10 20 30 + 987 octagonal 21 40 65 + 8640 odious 21 31 32 + 987654321 pancake 92 631 742 + 86321 panconsummate 10 20 21 + 721 pandigital 21 75 210 + 9876543210 partition 30 42 pentagonal 51 70 92 + 643210 pernicious 10 20 21 + 9876542 Perrin 10 51 90 853 persistent 9876543210 Pierpont 73 97 Poulet 8321 power 32 64 81 + 961 powerful 32 64 72 + 972 practical 20 30 32 + 9876540 prim.abundant 20 30 42 + 76542 prime 31 41 43 + 98765431 primorial 30 210 pronic 20 30 42 + 96410 Proth 41 65 81 + 8321 pseudoperfect 20 30 40 + 987654 rare 65 repfigit 61 75 742 repunit 21 31 40 + 87321 Ruth-Aaron 50 Saint-Exupery 60 self 20 31 42 + 976543210 semiprime 10 21 51 + 98754321 sliding 20 52 65 + 6410 Smith 85 94 654 + 98765432 Sophie Germain 41 53 83 + 986543 sphenic 30 42 70 + 98765421 square 64 81 841 961 star 73 541 9841 straight-line 210 321 420 + 9876543210 strobogrammatic 96 986 strong prime 41 71 97 + 98765431 super Niven 10 20 30 + 840 super-d 31 81 310 + 9876543 superabundant 60 720 840 tau 40 60 72 + 987654320 tetrahedral 10 20 84 85320 triangular 10 21 91 + 986310 tribonacci 81 trimorphic 51 75 76 + 8751 truncatable prime 31 43 53 + 87643 twin 31 41 43 + 98764321 uban 10 20 21 + 98 Ulam 53 62 72 + 9876532 unprimeable 320 510 530 + 9876542 untouchable 52 96 210 + 987652 upside-down 64 73 82 + 987654321 wasteful 20 30 40 + 9876543 weak prime 31 43 61 + 98764321 weird 70 76510 87430 + 975310 Wieferich 7651 Woodall 63 80 Zuckerman 432 Zumkeller 20 30 40 + 98760