[go: up one dir, main page]
Search a number
self or Colombian numbers
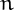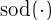A number  $n$  is a self number, or Colombian number if it cannot be written as  $x+\mathrm{sod}(x)$  where  $\mathrm{sod}(\cdot)$  denotes the sum of digits.

For example,  $25$  is not a self number because  $17+\mathrm{sod}(17)=17+1+7=25$, while  $20$  is a self number because there is not a number which added to its sum of digits gives  $20$.

D.R.Kaprekar, who introduced these numbers in 1949, gave the following test to determine quickly if a number is self.

Define the functions

\[ \begin{array}{lcl} d(n)& = & \mathrm{the\ number\ of\ digits\ of\ }n\,,\\[1mm] r(n)\vphantom{M^{M^M}a_{M_M}}&=& 1+({{(n-1)}\pmod9})\,, \\[1mm] h(n)&=&\left\{% \begin{array}{ll} r(n)/2&\mathrm{\ if\ }r(n)\mathrm{\ is\ even}\,,\\ (r(n)+9)/2&\mathrm{\ if\ }r(n)\mathrm{\ is\ odd}\,.\\ \end{array}\right.\\ \end{array} \]

Then a number  $n$  is self if, for every  $k=0,1,\dots,d(n)$, it holds

\[ \mathrm{sod}(|n-h(n)-9\cdot k|) \neq h(n)+9\cdot k\, \]
where  $|x|$  denotes the absolute value of  $x$.

The numbers which can be written in more than one way as  $x+\mathrm{sod}(x)$  are called junction numbers.

The first self numbers are 1, 3, 5, 7, 9, 20, 31, 42, 53, 64, 75, 86, 97, 108, 110, 121, 132, 143, 154, 165, 176 more terms

Below, the spiral pattern of self numbers up to  $250^2$. See the page on prime numbers for an explanation and links to similar pictures.

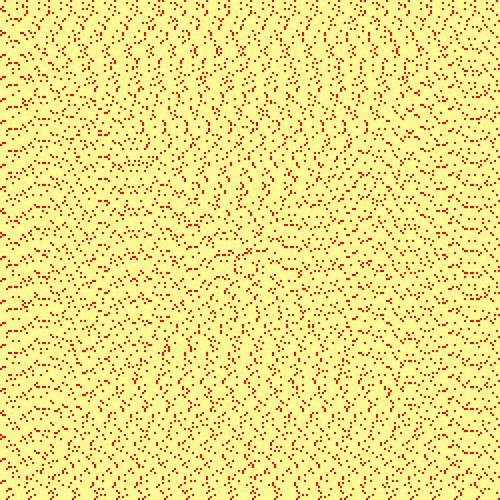
spiral pattern of self numbers

Pictorial representation of remainders (mod 2, 3, ...,11) frequency. For a table of values and more details click here
remainders of self numbers

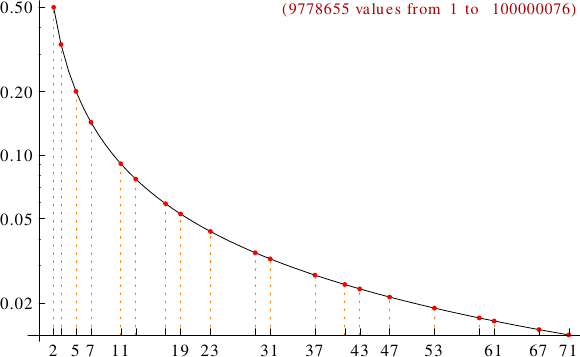
A graph displaying how many self numbers are multiples of the primes p from 2 to 71. In black the ideal line 1/p.
divisibility of self numbers
Useful links Mathworld, Self Number
Wikipedia, Self number
OEIS, Sequence A003052
Self numbers can also be... (you may click on names or numbers and on + to get more values)

a-pointer 2213 3089 4057 + 999996407 ABA 64 288 512 + 999849762 aban 20 31 42 + 1000000087 abundant 20 42 108 + 49999980 Achilles 108 288 648 + 999760328 admirable 20 42 222 + 99999726 alternating 121 143 165 + 989898969 amenable 20 53 64 + 999999972 amicable 1210 17296 66928 + 991581075 anti-perfect 244 133857 apocalyptic 222 312 411 + 29995 arithmetic 20 31 42 + 9999998 astonishing 3078 2006118 202272147 + 232728727 automorphic 9376 balanced p. 53 211 1223 + 999979397 bemirp 16001 198901 11816011 + 199800091 betrothed 75 16587 196664 + 987827555 binomial 20 165 378 + 999246160 brilliant 121 143 187 + 999999467 c.decagonal 31 211 80011 + 997790011 c.heptagonal 547 1772 4663 + 999220993 c.nonagonal 703 3403 4753 + 999246160 c.octagonal 121 6889 9409 + 999887641 c.pentagonal 31 681 951 + 998550526 c.square 613 1513 1985 + 999805045 c.triangular 31 64 514 + 999272866 cake 42 64 176 + 993213978 Carmichael 52633 334153 488881 + 958762729 Carol 261119 16769023 Catalan 42 132 9694845 Chen 31 53 211 + 99999827 compositorial 1728 congruent 20 31 53 + 9999998 constructible 20 64 255 + 855651072 cube 64 512 1728 + 973242271 Cullen 22529 106497 2228225 402653185 Cunningham 31 143 244 + 999761162 Curzon 53 86 165 + 199999749 cyclic 31 53 97 + 9999965 D-number 411 501 591 + 7042773 d-powerful 132 209 334 + 9999752 de Polignac 905 1087 1199 + 99999895 decagonal 637 5967 10660 + 999524032 deceptive 703 12403 52633 + 991565953 deficient 31 53 64 + 9999998 dig.balanced 42 75 108 + 199999874 double fact. 34459425 654729075 droll 6272 14080 304128 + 732168192 Duffinian 64 75 121 + 9999965 eban 42 64 2044 + 66066066 economical 31 53 64 + 19999999 emirp 31 97 389 + 199999661 emirpimes 143 187 413 + 99999794 enlightened 2560 23328 2317312 + 358722675 equidigital 31 53 64 + 19999999 eRAP 20 3438 13775 + 999164932 esthetic 121 323 345 + 989898789 Eulerian 15619 67108837 848090912 evil 20 53 75 + 999999983 fibodiv 75 244 323 + 984380344 Fibonacci 233 17711 121393 1346269 Friedman 121 2503 3864 + 995346 frugal 512 1458 1715 + 999990625 gapful 108 110 121 + 999999735 Gilda 110 good prime 53 97 569 + 199883539 happy 31 86 97 + 9999976 harmonic 1638 18600 55860 + 825120800 Harshad 20 42 108 + 999999926 heptagonal 1177 7756 10144 + 999270133 hex 547 817 2437 + 998366419 hexagonal 378 435 703 + 999112051 hoax 424 861 1111 + 99997915 Hogben 31 211 703 + 999160491 Honaker 457 2729 3067 + 999831103 house 28882 35555 78338 + 965131337 hungry 2401519 hyperperfect 10693 214273 1787917 + 972261181 iban 20 42 110 + 777770 iccanobiF 514 idoneal 42 165 312 + 760 impolite 64 512 65536 + 536870912 inconsummate 75 266 411 + 999978 insolite 11112 interprime 42 64 86 + 99999950 Jordan-Polya 64 288 512 + 905969664 Kaprekar 703 38962 142857 + 74747475 katadrome 20 31 42 + 976543210 Kynea 1087 Lehmer 255 435 703 + 999271663 Leyland 512 1649 6250 + 268436240 lonely 53 211 31428 + 20831427 Lucas 15127 39603 3010349 + 141422324 lucky 31 75 211 + 9999807 Lynch-Bell 132 312 648 + 9812376 m-pointer 12113 1111213 11264111 + 136211111 magic 1379 4641 6924 + 981258130 magnanimous 20 110 209 + 22600601 metadrome 345 356 367 + 12456789 modest 209 211 222 + 999776223 Moran 42 198 209 + 99999963 Motzkin 323 5798 142547559 narcissistic 92727 548834 472335975 nialpdrome 20 31 42 + 999999994 nonagonal 75 154 1491 + 999237889 nude 132 222 244 + 499999932 O'Halloran 20 oban 20 53 75 + 995 octagonal 176 3008 3201 + 999808096 odious 31 42 64 + 999999994 Ormiston 40213 48109 49279 + 999992537 palindromic 121 222 323 + 999939999 palprime 727 929 30403 + 998282899 pancake 121 154 211 + 999693256 panconsummate 20 31 53 + 413 pandigital 75 108 198 + 381366956 partition 42 176 490 + 541946240 pentagonal 176 782 1335 + 999918232 pernicious 20 31 42 + 9999998 Perrin 209 277 367 + 727653 Pierpont 97 2593 18433 plaindrome 222 233 244 + 888888999 Poulet 4371 15709 31417 + 997753901 power 64 121 400 + 999887641 powerful 64 108 121 + 999887641 practical 20 42 64 + 9999840 prim.abundant 20 42 222 + 99999726 prime 31 53 97 + 1000000087 primeval 1379 10379 100379 + 100234579 pronic 20 42 110 + 999350156 Proth 97 209 929 + 999915521 pseudoperfect 20 42 108 + 1000000 repdigit 222 1111 88888 + 555555555 repfigit 75 1537 3684 + 7913837 repunit 31 121 211 + 999160491 Rhonda 5664 15698 25284 + 977221215 Ruth-Aaron 154 714 1682 + 998141265 Saint-Exupery 97440 131820 245760 + 989475960 Sastry 226123528 self-describing 666666 10173133 10311733 + 42292924 semiprime 86 121 143 + 99999917 sliding 20 110 3157 + 250000004 Smith 121 378 648 + 99999050 Sophie Germain 53 233 659 + 999998903 sphenic 42 110 154 + 99999939 square 64 121 400 + 999887641 star 121 793 2773 + 990041221 straight-line 222 345 468 + 555555555 strobogrammatic 916 1111 6889 + 999888666 strong prime 97 277 367 + 99998231 subfactorial 14833 14684570 super Niven 20 110 400 + 888880800 super-d 31 310 334 + 9999919 tau 108 132 288 + 999999544 taxicab 13832 20683 64232 + 995125824 tetrahedral 20 165 10660 + 994856555 tetranacci 108 10671 76424 + 387559437 triangular 378 435 703 + 999246160 tribonacci 121415 223317 trimorphic 75 501 749 + 425781249 truncatable prime 31 53 97 + 995729173 twin 31 569 659 + 999996071 uban 20 31 42 + 1000000087 Ulam 53 97 209 + 10000109 undulating 121 323 424 + 797979797 unprimeable 512 514 536 + 9999965 untouchable 288 626 670 + 999842 upside-down 64 951 1199 + 699951114 vampire 104260 132430 135837 + 86139495 wasteful 20 42 75 + 9999998 weak prime 31 233 389 + 99999827 weakly prime 6173731 7469797 10564877 + 998839951 weird 7912 10570 23170 + 997348 Wieferich 17555 410787 18485415 + 518065605 Woodall 323 24575 114687 + 9961471 Zeisel 4505 5719 287979 + 995762585 Zuckerman 132 312 1111 + 997711344 Zumkeller 20 42 108 + 99980 zygodrome 222 1111 1122 + 999999555