[go: up one dir, main page]
Search a number
twin primes
Two primes are said to be twins if their difference is 2, like in the pairs (29, 31) or (977779797977, 977779797979).

So, a prime  $p$  is called twin prime if  $p+2$  or  $p-2$  is prime as well.

It is conjectured, but still not proved, that there are infinite twin primes.

Primes which do not belong to a twin pair are sometimes called isolated.

Probably all the even numbers greater than 4208 can be written as the sum of two twin primes.

The first twin pairs are (3, 5), (5, 7), (11, 13), (17, 19), (29, 31), (41, 43), (59, 61), (71, 73), (101, 103), (107, 109) more terms

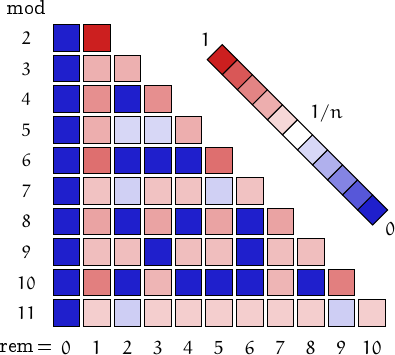
Pictorial representation of remainders (mod 2, 3, ...,11) frequency. For a table of values and more details click here
remainders of twin primes
Useful links Mathworld, Twin Primes
Wikipedia, Twin prime
OEIS, sequence A001097
Twin primes can also be... (you may click on names or numbers and on + to get more values)

a-pointer 11 13 + 999877519 999905971 aban 11 13 + 999000491 999000493 alt.fact. 19 101 + 4421 35899 alternating 29 41 + 989894747 989894749 amenable 13 17 + 999998957 999999193 apocalyptic 823 857 + 29879 29881 arithmetic 11 13 + 9999971 9999973 bemirp 1061 1091 + 198998881 199680881 c.decagonal 11 31 + 997507501 999768701 c.heptagonal 43 71 + 985430671 990252271 c.pentagonal 31 181 + 989975251 995654731 c.square 13 41 + 996588013 999983921 c.triangular 19 31 + 997802209 998730919 Chen 11 13 + 99999539 99999587 congruent 13 29 + 9999047 9999973 constructible 17 65537 Cunningham 17 31 + 975063077 995402501 Curzon 29 41 + 199981889 199982969 cyclic 11 13 + 9999971 9999973 d-powerful 43 283 + 9965729 9972583 de Polignac 149 599 + 99995669 99996131 deficient 11 13 + 9999971 9999973 dig.balanced 11 19 + 199992449 199994369 economical 11 13 + 19999547 19999549 emirp 13 17 + 199999307 199999309 equidigital 11 13 + 19999547 19999549 esthetic 43 101 + 343234321 345434567 Eulerian 11 65519 478271 evil 17 29 + 999998959 999999193 fibodiv 19 61 + 1999 2087 Fibonacci 13 Friedman 347 12107 + 976559 995341 Gilda 29 good prime 11 17 + 199884017 199968539 happy 13 19 + 9999161 9999929 hex 19 61 + 992682871 995304031 Hogben 13 31 + 998338813 998907631 Honaker 1049 1091 + 999611309 999815041 house 271 hungry 17 iban 11 17 + 777011 777421 iccanobiF 13 idoneal 13 inconsummate 431 461 + 998027 999331 Jacobsthal 11 43 + 174763 715827883 junction 101 103 + 99999257 99999259 katadrome 31 41 + 9875321 98764321 Leyland 17 Lucas 11 29 199 521 lucky 13 31 + 9998971 9999049 m-pointer 61 1231 + 111316111 311221111 magnanimous 11 29 + 8608081 228440489 metadrome 13 17 + 1235789 1245689 modest 13 19 + 998047619 999311111 nialpdrome 11 31 + 999997771 999998641 nude 11 oban 11 13 + 859 883 odious 11 13 + 999998957 999999191 Ormiston 1931 25031 + 999962479 999980897 palindromic 11 101 + 999434999 999454999 palprime 11 101 + 999434999 999454999 pancake 11 29 + 994423907 998709779 panconsummate 11 31 + 1093 1291 pandigital 11 19 partition 11 101 pernicious 11 13 + 9999971 9999973 Perrin 17 29 Pierpont 13 17 + 63700993 169869313 plaindrome 11 13 + 668999999 677888999 prime 11 13 + 999999191 999999193 primeval 13 107 + 1002347 10034579 Proth 13 17 + 995033089 995622913 repdigit 11 repfigit 19 61 197 repunit 13 31 + 998338813 998907631 self 31 569 + 999994651 999996071 self-describing 10153331 16331531 + 33151231 33151931 sliding 11 29 101 641 Sophie Germain 11 29 + 999998141 999999191 star 13 73 + 967968613 972241021 straight-line 76543 strobogrammatic 11 101 + 668609899 690181069 strong prime 11 17 + 99999539 99999587 super-d 19 31 + 9998969 9999931 tetranacci 29 tribonacci 13 149 trimorphic 31249 281249 truncatable prime 13 17 + 993946997 998966653 uban 11 13 + 97000019 97000021 Ulam 11 13 + 9998203 9999161 undulating 101 151 + 95959 1212121 upside-down 19 73 + 99619411 99955111 weak prime 13 19 + 99999541 99999589 weakly prime 294001 1062599 + 994125569 998839951 Wieferich 1093 Woodall 17 191 3124999 Zuckerman 11 zygodrome 11 11777 + 999922111 999922211