A number is a zygodrome in a given base 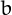 (usually 10)
if in that base it is made of nontrivial runs of identical digits.
(usually 10)
if in that base it is made of nontrivial runs of identical digits.
![\[
c(n)=2\cdot c(n - 1) + 8\cdot c(n - 2) - 9\cdot c(n - 3)\,.
\]](https://speed.lescigales.org/xypor/index.php?q=aHR0cHM6Ly93d3cubnVtYmVyc2FwbGVudHkuY29tL3NldC96eWdvZHJvbWUvcGljLjcucG5n)
I call these numbers zygodromes because digits appear at least in pairs and the Greek root for pair is zygo-.
For example, 112277, 44444333 and 55500111 are all zygodromes in base 10.
The total number of zygodromes in base 10 with at most
digits is given by the recurrence defined by
,
,
and
The sum of the reciprocals of zygodromes converges to a number approximatively equal to 0.3164971.
The prime is the only known zygodrome prime whose index is a zygodrome as well.
The first zygodromes (in base 10) are 11, 22, 33, 44, 55, 66, 77, 88, 99, 111, 222, 333, 444, 555, 666, 777, 888, 999, 1100, 1111, 1122, 1133 more terms
Pictorial representation of remainders (mod 2, 3, ...,11) frequency. For a table of values and more details click here

A graph displaying how many zygodromes are multiples of the primes p from 2 to 71. In black the ideal line 1/p.

Useful links
OEIS, Sequence A033023
Zygodromes can also be... (you may click on names or numbers and on + to get more values)
a-pointer
11
4443311
33322111
+
9922288811
ABA
447722888
11000004488
22244466888
+
8877886668800
aban
11
22
33
+
999999000999
abundant
66
88
222
+
44999988
Achilles
665500
5556600
6655000
+
44885588800000
admirable
66
88
222
+
99933222
amenable
33
44
77
+
999999988
apocalyptic
222
666
1133
+
22999
arithmetic
11
22
33
+
9999999
astonishing
3388
balanced p.
3355577
5555777
7722277
+
9993355511
binomial
55
66
666
+
5553333000111
brilliant
22999
55577
1166111
+
999997777
c.decagonal
11
22111
445511
+
884441155522111
c.heptagonal
22
4411
669922
+
11662266115522
c.nonagonal
55
111221155
c.triangular
6644778888844
8882229992266
55777555332244
+
110055449944444
Chen
11
11177
11777
+
99955577
congruent
22
55
77
+
9999999
Cunningham
33
99
999
+
557755556688800
Curzon
33
1133
1166
+
119999333
cyclic
11
33
77
+
9999977
D-number
33
111
11199
+
6666999
d-powerful
333
2244
22333
+
9933222
de Polignac
1199
7799
44111
+
99996677
decagonal
1117777
22994422
6663334455
+
880000077224422
deceptive
7777
11111
1111111
+
11188888777
deficient
11
22
33
+
9999999
dig.balanced
11
44
99
+
118800444
Duffinian
55
77
111
+
9999977
eban
44
66
44000
+
66000066000066
economical
11
111
6655
+
11999933
emirp
11777
77711
1133333
+
119988899
emirpimes
1177
7711
7799
+
99999911
enlightened
119911
equidigital
11
111
6655
+
11999933
eRAP
2255
66661111
336655777
Eulerian
11
66
evil
33
66
77
+
999999988
fibodiv
114488
Fibonacci
55
Friedman
116655
117755
117777
+
449955
frugal
1100000
1113344
1118866
+
999666111
gapful
1100
1111
1155
+
99999999900
good prime
11
11777
33311
+
117770011
happy
44
888
1122
+
9998877
Harshad
111
222
333
+
9999999666
heptagonal
55
1177
11122
+
881118800088111
hex
1188811
2200777
661166611
+
552299998822111
hexagonal
66
1188111
2200066611
+
558822550004400
highly composite
554400
hoax
22
1111
3344
+
99991155
Hogben
111
33777110011
77333770011
+
2255660055111
Honaker
22277
77711
1144499
+
999112211
iban
11
22
44
+
777777
idoneal
22
33
88
inconsummate
1166
3311
4466
+
999933
insolite
111
111111111
interprime
99
111
1100
+
99996633
Jacobsthal
11
junction
111
3322
4422
+
99999966
Kaprekar
55
99
999
+
999999999999999
Lehmer
1111
4411
11155
+
887755665511
Lucas
11
lucky
33
99
111
+
9999555
magic
111
665555
665500005500
665500000055000
magnanimous
11
modest
111
222
333
+
1199999999
Moran
111
222
333
+
99966699
nialpdrome
11
22
33
+
999999999999999
nonagonal
111
5500
6666
+
990011222550099
nude
11
22
33
+
449996688
O'Halloran
44
oban
11
33
55
+
999
octagonal
1133002200
113300220033
2222669966000
+
222266996600033
odious
11
22
44
+
999999999
palindromic
11
22
33
+
999999999999999
palprime
11
1177711
7722277
+
999955444559999
pancake
11
22
1177
+
881155771115566
panconsummate
11
77
pandigital
11
99
33555
+
339955500
partition
11
22
77
pentagonal
22
8855
55777
+
88844444113322
pernicious
11
22
33
+
9999988
Perrin
22
plaindrome
11
22
33
+
999999999999999
power
7744
774400
77440000
+
8844449977444
powerful
7744
665500
774400
+
992255544007744
practical
66
88
666
+
9999900
prim.abundant
66
88
222
+
99933222
prime
11
11177
11777
+
999999988811
pronic
1122
4422
9900
+
99999990000000
Proth
33
8833
11777
+
222554488833
pseudoperfect
66
88
222
+
999999
repdigit
11
22
33
+
999999999999999
repunit
111
1111
11111
+
111111111111111
Rhonda
333355511122
Ruth-Aaron
77
2299
8855
+
449990004477
Saint-Exupery
555660000
555660000000
554444445555000
555660000000000
self
222
1111
1122
+
999999555
self-describing
22
4444
224444
+
88888888666666
semiprime
22
33
55
+
99999911
sliding
11
1100
11000
+
110000000000000
Smith
22
666
1111
+
99991155
Sophie Germain
11
44111
77711
+
9999988811
sphenic
66
222
555
+
99999922
square
7744
774400
77440000
+
992255544007744
star
220033
771133
332255533
77111779933
straight-line
111
222
333
+
999999999999999
strobogrammatic
11
88
111
+
999999888666666
strong prime
11
11777
22277
+
99955577
subfactorial
44
super Niven
1100
2200
3300
+
44880000000
super-d
333
1188
3322
+
9999666
superabundant
554400
tau
88
444
22000
+
999992244
tetrahedral
55228866
1188887700
triangular
55
66
666
+
558822550004400
tribonacci
44
trimorphic
99
999
9999
+
999999999999999
twin
11
11777
22111
+
999922211
uban
11
22
33
+
99000099000099
Ulam
11
77
99
+
9999999
unprimeable
1144
5544
6622
+
9999988
untouchable
88
3366
3388
+
999966
upside-down
55
555
1199
+
999999555111111
vampire
44995500
2266335544
5522776600
wasteful
22
33
44
+
9999999
weak prime
11177
22111
44777
+
99955111
weakly prime
77755114477
weird
776644
Woodall
99999999999
11999999999999
Zeisel
336611
Zuckerman
11
111
1111
+
3311331111
Zumkeller
66
88
222
+
99988