tetranacci numbers

Tetranacci numbers are defined by the recurrence 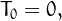$T_0=0,$

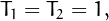
and $T_n=T_{n-1}+T_{n-2}+T_{n-3}+T_{n-4}$ for $n\ge4$ , so are similar to Fibonacci numbers, but at each step we add the previous 4 terms.

The first tetranacci numbers are 1, 1, 2, 4, 8, 15, 29, 56, 108, 208, 401, 773, 1490, 2872, 5536, 10671, 20569, 39648, 76424, 147312 more terms

Pictorial representation of remainders (mod 2, 3, ...,11) frequency. For a table of values and more details click here

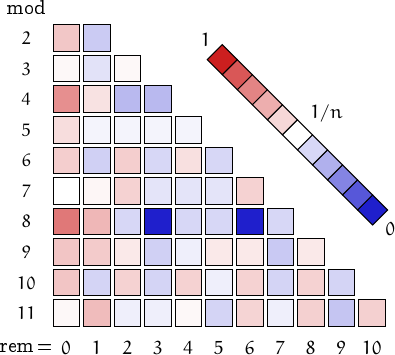
A graph displaying how many tetranacci numbers are multiples of the primes p from 2 to 71. In black the ideal line 1/p.

Useful links Mathworld, Tetranacci Number
Wikipedia, Generalizations of Fibonacci numbers
OEIS, Sequence A000078

Tetranacci numbers can also be... (you may click on names or numbers and on + to get more values)

aban 15 29 56 108 208 401 773 abundant 56 108 208 39648 147312 2033628 3919944 28074040 Achilles 108 admirable 56 alternating 29 56 1490 amenable 29 56 108 208 401 773 2872 5536 20569 39648 + 54114452 104308960 201061985 387559437 apocalyptic 1490 2872 10671 20569 arithmetic 15 29 56 401 773 2872 10671 20569 39648 147312 + 1055026 2033628 3919944 7555935 astonishing 15 Bell 15 binomial 15 56 brilliant 15 cake 15 Chen 29 401 congruent 15 29 56 208 773 2872 10671 39648 76424 147312 1055026 2033628 7555935 constructible 15 Cunningham 15 401 Curzon 29 cyclic 15 29 401 773 10671 20569 D-number 15 10671 283953 d-powerful 39648 2033628 de Polignac 14564533 deficient 15 29 401 773 1490 2872 5536 10671 20569 76424 283953 547337 1055026 7555935 dig.balanced 15 56 108 1490 2872 54114452 double fact. 15 Duffinian 20569 283953 547337 eban 56 economical 15 29 401 773 10671 20569 283953 547337 14564533 emirpimes 15 10671 547337 equidigital 15 29 401 773 10671 20569 283953 547337 14564533 esthetic 56 evil 15 29 108 401 773 1490 2872 20569 76424 147312 + 3919944 28074040 104308960 747044834 gapful 108 1490 147312 28074040 104308960 1439975216 10312882481 Gilda 29 good prime 29 happy 208 283953 1055026 7555935 Harshad 108 147312 hexagonal 15 hoax 2872 iban 401 773 147312 idoneal 15 inconsummate 147312 547337 interprime 15 56 108 283953 7555935 28074040 junction 208 1055026 2033628 7555935 14564533 28074040 Lehmer 15 Lucas 29 lucky 15 10671 Lynch-Bell 15 magic 15 magnanimous 29 56 401 metadrome 15 29 56 modest 29 nialpdrome 773 nude 15 oban 15 29 56 773 odious 56 208 5536 10671 39648 547337 1055026 2033628 7555935 14564533 54114452 201061985 387559437 pancake 29 56 panconsummate 15 pandigital 15 108 1490 partition 15 56 pernicious 56 208 5536 39648 547337 1055026 2033628 Perrin 29 plaindrome 15 29 56 powerful 108 practical 56 108 208 39648 147312 prim.abundant 56 prime 29 401 773 5350220959 pronic 56 pseudoperfect 56 108 208 39648 147312 repunit 15 Ruth-Aaron 15 self 108 10671 76424 283953 387559437 semiprime 15 10671 20569 283953 547337 14564533 sliding 29 Sophie Germain 29 sphenic 1490 strong prime 29 super-d 10671 20569 1055026 tau 56 108 2872 39648 tetrahedral 56 triangular 15 truncatable prime 29 773 twin 29 uban 15 29 56 unprimeable 208 39648 1055026 2033628 7555935 untouchable 39648 wasteful 56 108 208 1490 2872 5536 39648 76424 147312 1055026 2033628 3919944 7555935 weak prime 401 773 Zuckerman 15 Zumkeller 56 108 208 39648