Leyland numbers

A number

is a Leyland number if it can be written as $a^b+b^a$

, with

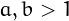1$">.

For example, 368 is a Leyland number because $368=5^3+3^5$ .

Leyland numbers have been studied because some of them are pretty large primes, like $8656^{2929} + 2929^{8656}$ (30008 digits), or $314738^9 + 9^{314738}$ (300337 digits).

The first Leyland numbers are 8, 17, 32, 54, 57, 100, 145, 177, 320, 368, 512, 593, 945, 1124, 1649, 2169, 2530, 4240, 5392, 6250, 7073, 8361 more terms

Pictorial representation of remainders (mod 2, 3, ...,11) frequency. For a table of values and more details click here

A graph displaying how many Leyland numbers are multiples of the primes p from 2 to 71. In black the ideal line 1/p.

Useful links Wikipedia, Leyland number
OEIS, Sequence A076980

Leyland numbers can also be... (you may click on names or numbers and on + to get more values)

ABA 32 512 93312 33554432 774840978 20000000000 aban 17 32 54 57 100 145 + 20000000000 abundant 54 100 320 368 945 2530 + 14352282 Achilles 93312 20000000000 17832200896512 admirable 54 368 945 alternating 32 54 145 945 2169 8361 + 69632 amenable 17 32 57 100 145 177 + 536871753 apocalyptic 2530 4240 5392 6250 7073 8361 + 23401 arithmetic 17 54 57 145 177 593 + 9865625 balanced p. 593 32993 brilliant 1649 c.square 145 Chen 17 32993 congruent 54 145 320 368 1649 2530 + 9865625 constructible 17 32 320 512 65792 69632 + 281479271677952 cube 512 Cunningham 17 145 Curzon 54 593 1649 2169 18785 131361 + 177264449 cyclic 17 145 177 593 1649 7073 + 4785713 D-number 57 177 131361 423393 d-powerful 4240 262468 2097593 de Polignac 1649 32993 deficient 17 32 57 145 177 512 + 9865625 dig.balanced 177 2169 2530 60049 8389137 10609137 double fact. 945 Duffinian 32 57 100 512 1649 2169 + 8389137 eban 32 54 economical 17 32 145 177 320 512 + 16797952 emirp 17 emirpimes 177 7073 131361 equidigital 17 32 145 177 320 593 + 16777792 esthetic 32 54 Eulerian 57 evil 17 54 57 177 320 368 + 774840978 Friedman 93312 262468 533169 frugal 512 6250 93312 1647086 10609137 16797952 + 774840978 gapful 100 4240 16580 1048976 1058576 1596520 + 20000000000 good prime 17 593 happy 32 100 320 368 18785 60049 4194788 Harshad 54 100 320 512 2530 4240 + 2179768320 Hogben 57 house 32 hungry 17 iban 17 100 177 320 1124 4240 + 23401 idoneal 57 177 impolite 32 512 33554432 inconsummate 945 1649 20412 65792 131361 423393 Jordan-Polya 32 512 93312 33554432 17832200896512 junction 20412 94932 8389137 33554432 67109540 katadrome 32 54 320 lucky 131361 268705 magic 4294968320 magnanimous 32 512 metadrome 17 57 145 368 Moran 16580 nialpdrome 32 54 100 320 20000000000 nude 1124 93312 94932 oban 17 57 320 368 512 593 odious 32 100 145 512 4240 7073 + 387426321 panconsummate 54 57 pandigital 177 pentagonal 145 pernicious 17 100 145 320 4240 7073 + 9865625 Perrin 17 Pierpont 17 plaindrome 17 57 145 177 368 1124 power 32 100 512 33554432 powerful 32 100 512 93312 33554432 20000000000 17832200896512 practical 32 54 100 320 368 512 + 1941760 prim.abundant 368 945 2530 65792 prime 17 593 32993 2097593 8589935681 pronic 65792 4295032832 Proth 17 57 145 177 pseudoperfect 54 100 320 368 945 2530 + 94932 repunit 57 self 512 1649 6250 131361 10609137 268436240 semiprime 57 145 177 1649 7073 23401 + 43050817 sliding 20000000000 Smith 67137425 Sophie Germain 593 sphenic 60049 178478 268705 397585 524649 8389137 square 100 strong prime 17 2097593 super Niven 100 20000000000 super-d 2169 533169 1048976 1058576 1596520 tau 4240 20412 268436240 truncatable prime 17 593 twin 17 uban 17 32 57 20000000000 Ulam 57 145 177 2012174 unprimeable 320 512 16580 65792 69632 178478 + 1058576 untouchable 2530 16580 69632 93312 94932 178478 262468 upside-down 1649 wasteful 54 57 100 368 945 1124 + 8389137 Woodall 17 Zuckerman 93312 Zumkeller 54 320 368 945 2530 4240 + 94932