[go: up one dir, main page]
Search a number
tribonacci numbers
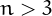Tribonacci numbers are defined by the recurrence  $T_1=T_2=1$,  $T_3=2$  and  $T_n=T_{n-1}+T_{n-2}+T_{n-3}$  for  $n3$">, so are similar to Fibonacci numbers, but each time we add the previous 3 terms.

The first tribonacci numbers are 1, 1, 2, 4, 7, 13, 24, 44, 81, 149, 274, 504, 927, 1705, 3136, 5768, 10609, 19513, 35890, 66012 more terms

Pictorial representation of remainders (mod 2, 3, ...,11) frequency. For a table of values and more details click here
remainders of tribonacci numbers

A graph displaying how many tribonacci numbers are multiples of the primes p from 2 to 71. In black the ideal line 1/p.
divisibility of tribonacci numbers
Useful links Mathworld, Tribonacci Number
Wikipedia, Generalizations of Fibonacci numbers
OEIS, Sequence A000073
Tribonacci numbers can also be... (you may click on names or numbers and on + to get more values)

a-pointer 13 ABA 24 81 aban 13 24 44 81 149 274 504 927 abundant 24 504 3136 5768 66012 8646064 admirable 24 alternating 81 149 274 927 amenable 13 24 44 81 149 504 1705 3136 5768 10609 + 53798080 98950096 181997601 334745777 apocalyptic 927 5768 10609 19513 arithmetic 13 44 149 504 1705 5768 10609 19513 66012 121415 410744 2555757 8646064 brilliant 10609 c.octagonal 81 10609 c.square 13 c.triangular 274 Chen 13 149 compositorial 24 congruent 13 24 149 504 927 1705 66012 121415 223317 410744 755476 2555757 8646064 constructible 24 Cunningham 24 Curzon 81 cyclic 13 149 121415 d-powerful 24 de Polignac 149 deficient 13 44 81 149 274 927 1705 10609 19513 35890 + 755476 1389537 2555757 4700770 dig.balanced 44 149 10609 223317 8646064 Duffinian 81 927 1705 3136 10609 19513 223317 1389537 eban 44 economical 13 81 149 3136 10609 121415 223317 15902591 emirp 13 149 equidigital 13 81 149 3136 121415 15902591 eRAP 24 evil 24 149 504 927 1705 35890 121415 410744 2555757 4700770 + 98950096 181997601 334745777 615693474 factorial 24 fibodiv 149 Fibonacci 13 frugal 10609 223317 gapful 19513 223317 29249425 good prime 149 happy 13 44 Harshad 24 81 504 19513 8646064 29249425 53798080 heptagonal 81 highly composite 24 hoax 274 2555757 Hogben 13 iban 24 44 274 223317 410744 iccanobiF 13 idoneal 13 24 inconsummate 927 223317 interprime 81 274 410744 53798080 Jordan-Polya 24 junction 10609 66012 katadrome 81 lucky 13 927 1705 10609 Lynch-Bell 24 metadrome 13 24 149 modest 13 927 19513 nialpdrome 44 81 nonagonal 24 nude 24 44 O'Halloran 44 oban 13 927 odious 13 44 81 274 3136 5768 10609 19513 66012 223317 + 1389537 15902591 29249425 53798080 palindromic 44 panconsummate 24 81 pernicious 13 24 44 81 274 3136 5768 10609 19513 66012 1389537 Pierpont 13 plaindrome 13 24 44 149 power 81 3136 10609 powerful 81 3136 10609 practical 24 504 3136 5768 8646064 prime 13 149 primeval 13 Proth 13 81 pseudoperfect 24 504 3136 5768 66012 repdigit 44 repunit 13 Ruth-Aaron 24 self 121415 223317 semiprime 274 10609 Smith 274 sphenic 1705 19513 121415 4700770 square 81 3136 10609 star 13 strong prime 149 subfactorial 44 super Niven 24 super-d 81 10609 410744 superabundant 24 tau 24 504 66012 410744 trimorphic 24 truncatable prime 13 twin 13 149 uban 13 81 Ulam 13 927 5768 unprimeable 5768 66012 121415 untouchable 3136 5768 66012 wasteful 24 44 274 504 927 1705 5768 19513 35890 66012 + 1389537 2555757 4700770 8646064 weak prime 13 Zuckerman 24 Zumkeller 24 504 5768 66012 zygodrome 44