[go: up one dir, main page]
Search a number
junction numbers
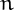A number  $n$  is a junction number if it can be written as  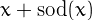$x+\mathrm{sod}(x)$  for at least two  $x$, where  $\mathrm{sod}(\cdot)$  denotes the sum of digits.

For example,  $818$  is a junction number because it has two generators,  $796$  and  $805$. Indeed

\[796+7+9+6 = 805+8+0+5 = 818.\]

The numbers which cannot be written at all as  $x+\mathrm{sod}(x)$  are called self numbers.

The following table reports the number of junction numbers up to  $10^k$  for  $k=3,4,\dots,10$.

up to103 104105106 1071081091010
# 81954974797740977733977772697777719977777712

The following table reports the smallest junction number with  $k$  generators, for for  $k=2,3,\dots,7$.

 $k$junction number
 $2$  $101$ 
 $3$  $10000000000001$
 $4$ $1000000000000000000000102$
 $5$ $10^{1111111111124} + 102$
 $6$ $10^{2222222222224} + 10^{13}+2$
 $7$ $10^{(10^{24} + 10^{13} + 115) / 9} + 10^{13} + 2$
(The last entries in the table are from Max Alekseyev who has found all the terms up to the 100th!)

The first junction numbers are 101, 103, 105, 107, 109, 111, 113, 115, 117, 202, 204, 206, 208, 210, 212, 214, 216, 218, 303, 305, 307, 309, 311, 313, 315, 317, 319, 404, 406, 408, 410, 412, 414, 416, 418, 420, 505, 507 more terms

Below, the curious spiral pattern of junction numbers up to  $250^2$. See the page on prime numbers for an explanation and links to similar pictures.

spiral pattern of junction numbers

Pictorial representation of remainders (mod 2, 3, ...,11) frequency. For a table of values and more details click here
remainders of junction numbers

A graph displaying how many junction numbers are multiples of the primes p from 2 to 71. In black the ideal line 1/p.
divisibility of junction numbers
Useful links Mathworld, Self Number
Wikipedia, Self number
OEIS, Sequence A230094
OEIS, Sequence A006064
Junction numbers can also be... (you may click on names or numbers and on + to get more values)

a-pointer 101 103 1409 + 99951043 ABA 1024 1215 1922 + 99828450 aban 101 103 105 + 99000943 abundant 204 208 210 + 49999860 Achilles 5324 14112 16928 + 99715239 admirable 606 618 812 + 99999956 alt.fact. 101 3301819 alternating 101 103 105 + 98989058 amenable 101 105 109 + 99999968 amicable 2620 5020 63020 + 97580944 apocalyptic 218 610 612 + 29936 arithmetic 101 103 105 + 9999961 astonishing 204 216 1301613 7103835 automorphic 90625 890625 2890625 balanced p. 6317 6323 7823 + 99986251 Bell 27644437 betrothed 312620 1348935 1763019 + 88567059 binomial 105 210 406 + 99892045 brilliant 319 517 713 + 98962343 c.decagonal 101 911 2311 + 99926851 c.heptagonal 3928 6931 10018 + 99524447 c.nonagonal 406 820 3916 + 99892045 c.octagonal 2025 5625 7225 + 99860049 c.pentagonal 3516 15016 17016 + 99713851 c.square 113 313 925 + 99870845 c.triangular 109 1219 1306 + 99890641 cake 6018 9920 30914 + 91895351 Carmichael 126217 552721 997633 + 99830641 Carol 65023 Catalan 208012 Chen 101 107 109 + 99999257 congruent 101 103 109 + 9999959 constructible 204 408 816 + 84215045 cube 216 4913 13824 + 99252847 Cullen 491521 Cunningham 101 511 513 + 99897345 Curzon 105 113 210 + 99999869 cyclic 101 103 107 + 9999959 D-number 111 303 309 + 7042227 d-powerful 1306 2315 2517 + 9997442 de Polignac 509 1207 1211 + 99999857 decagonal 3510 12825 17226 + 99865045 deceptive 10001 11111 22321 + 99830641 deficient 101 103 105 + 9999961 dig.balanced 202 204 210 + 95584540 double fact. 105 645120 2027025 10321920 droll 39424 2838528 33161216 + 53215232 Duffinian 111 115 305 + 9999959 eban 2002 2004 2006 + 66066036 economical 101 103 105 + 19999946 emirp 107 113 311 + 99999259 emirpimes 115 319 511 + 99999853 enlightened 2238728 equidigital 101 103 105 + 19999946 eRAP 1104 7625 20220 + 99979346 esthetic 101 210 212 + 89898765 Eulerian 1013 2416 88234 + 13824739 evil 101 105 111 + 99999968 fibodiv 305 911 11709 + 87374946 Fibonacci 610 75025 Friedman 216 1022 1024 + 996543 frugal 1024 1215 4913 + 99980041 gapful 105 315 1001 + 99999360 Gilda 35422 18633637 92078232 Giuga 1722 good prime 101 307 311 + 99904439 happy 103 109 208 + 9999945 harmonic 14303520 23963940 52141320 Harshad 111 117 204 + 99999966 heptagonal 616 1918 5221 + 99562336 hex 721 919 2107 + 99584647 hexagonal 2016 3003 6216 + 99595441 highly composite 27720 166320 720720 + 8648640 hoax 202 319 517 + 99999150 Hogben 111 307 507 + 99870043 Honaker 2719 4723 5009 + 99954551 house 1716 7617 112618 + 99402731 hungry 37929 33662541 hyperperfect 19521 495529 1055833 + 94472041 iban 101 103 107 + 777744 iccanobiF 12815 idoneal 105 210 408 1320 impolite 1024 33554432 inconsummate 216 416 521 + 999938 insolite 111 interprime 105 111 309 + 99999348 Jacobsthal 349525 44739243 Jordan-Polya 216 1024 1920 + 94371840 Kaprekar 22222 95121 466830 + 86358636 katadrome 210 410 420 + 9876543 Lehmer 511 1417 1615 + 99948451 Leyland 20412 94932 8389137 + 67109540 lonely 58831 370313 370315 + 20831428 Lucas 521 lucky 105 111 115 + 9999961 Lynch-Bell 216 315 412 + 9617328 m-pointer 2111 13121 15121 + 23311111 magic 111 505 2925 + 72766051 magnanimous 101 1001 1112 + 71553536 modest 103 109 111 + 99921951 Moran 111 117 511 + 99997362 Motzkin 15511 narcissistic 24678050 nialpdrome 111 210 311 + 99999970 nonagonal 111 204 14625 + 99679149 nude 111 115 212 + 99999162 O'Halloran 204 420 oban 303 305 307 + 925 octagonal 408 1825 5720 + 99971041 odious 103 107 109 + 99999970 Ormiston 19031 25013 34613 + 99940639 palindromic 101 111 202 + 94999949 palprime 101 313 919 + 9492949 pancake 1712 2017 2212 + 99934454 panconsummate 721 pandigital 210 216 1014 + 95584540 partition 101 3718 12310 + 3087735 pentagonal 117 210 715 + 99996755 pernicious 103 107 109 + 9999961 Perrin 4610 6107 313007 + 49405543 Pierpont 109 2917 786433 plaindrome 111 113 115 + 33333334 Poulet 8321 11305 126217 + 99830641 power 216 1024 2025 + 99880036 powerful 216 1024 2025 + 99880036 practical 204 208 210 + 9999858 prim.abundant 606 618 812 + 99999956 prime 101 103 107 + 99999259 primeval 107 113 1013 primorial 210 pronic 210 420 812 + 99630342 Proth 113 513 1217 + 99778561 pseudoperfect 204 208 210 + 999950 repdigit 111 11111 22222 + 22222222 repfigit 1104 2208 86935 355419 repunit 111 307 507 + 99870043 Rhonda 56718 95232 259333 + 94265232 Ruth-Aaron 105 715 1520 + 99143045 Saint-Exupery 16320 30720 65520 + 98672640 Sastry 715 2066115 self-describing 444422 10173331 10193331 + 42292429 semiprime 111 115 202 + 99999857 sliding 101 925 1001 + 97758650 Smith 202 319 517 + 99999160 Sophie Germain 113 509 719 + 99996749 sphenic 105 406 410 + 99999962 square 1024 2025 4624 + 99880036 star 2521 5221 12421 + 99951853 straight-line 111 210 420 + 22222222 strobogrammatic 101 111 808 + 99888866 strong prime 101 107 307 + 99999257 super Niven 204 210 408 + 93000060 super-d 105 107 319 + 9999753 superabundant 27720 166320 720720 + 8648640 tau 204 612 808 + 99999760 taxicab 65728 525824 1061424 + 97867441 tetrahedral 816 2925 16215 + 99846044 tetranacci 208 1055026 2033628 + 28074040 triangular 105 210 406 + 99892045 tribonacci 10609 66012 trimorphic 5625 90625 890625 2890625 truncatable prime 113 311 313 + 99187547 twin 101 103 107 + 99999259 uban 1000001 1000003 1000005 + 99000070 Ulam 206 309 319 + 10000041 undulating 101 202 212 + 49494949 unprimeable 204 206 208 + 9999955 untouchable 206 210 216 + 999952 upside-down 3827 4826 5825 + 96991141 vampire 102510 105210 116725 + 94619952 wasteful 117 202 204 + 9999961 weak prime 103 109 113 + 99999259 weakly prime 2474431 7982543 8353427 + 99778351 weird 11410 12110 16030 + 991130 Wieferich 14209 136929 2053935 57562845 Woodall 15624 279935 491519 97656249 Zeisel 105 1419 31929 + 79796761 Zuckerman 111 115 212 + 98224128 Zumkeller 204 208 210 + 99840 zygodrome 111 3322 4422 + 99999966