[go: up one dir, main page]
Search a number
Eulerian numbers
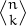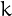The Eulerian number  $A(n,k)$, often denoted with  $\Eul{n}{k}$  is the number of permutations of the numbers  $\{1,2,\dots,n\}$  in which  $k$  elements are greater than the previous element, i.e., the number of permutations with  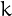$k$  ascents.

For example, among the  $4!=24$  permutations of  $\{1,2,3,4\}$, there are  $A(4,2)=11$  permutations with 2 ascents, like  $\{1, 2, 4, 3\}$, and  $\{3, 4, 1, 2\}$.

Eulerian numbers are given by the formula

\[ \Eul{n}{k}=\sum_{i=0}^{k+1}(-1)^i(k-i+1)^n{{n+1}\choose i}\,, \]
and are involved in many indentities, like
\[ n!=\sum_{k=0}^{n}\Eul{n}{k}\quad\mathrm{and}\quad x^n = \sum_{k=0}^{n-1}\Eul{n}{k}{{x+k}\choose n}\,. \]

The first distinct Eulerian numbers are 1, 4, 11, 26, 57, 66, 120, 247, 302, 502, 1013, 1191, 2036, 2416, 4083, 4293, 8178, 14608, 15619, 16369, 32752, 47840, 65519, 88234, 131054, 152637, 156190 more terms

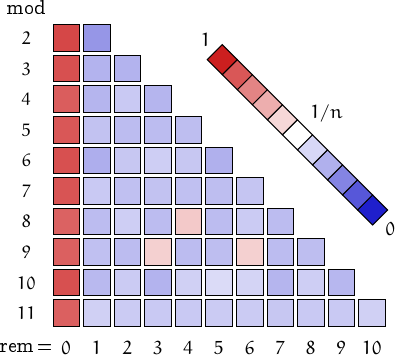
Pictorial representation of remainders (mod 2, 3, ...,11) frequency. For a table of values and more details click here
remainders of Eulerian numbers

A graph displaying how many Eulerian numbers are multiples of the primes p from 2 to 71. In black the ideal line 1/p.
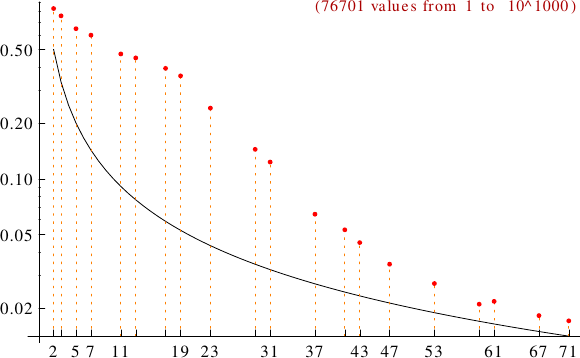
divisibility of Eulerian numbers
Useful links Wikipedia, Eulerian number
Mathworld, Eulerian Number
OEIS, Sequence A008292
Eulerian numbers can also be... (you may click on names or numbers and on + to get more values)

a-pointer 11 aban 11 26 57 66 120 247 302 502 abundant 66 120 8178 14608 32752 47840 131054 524268 1479726 + 33554406 admirable 66 120 alternating 16369 amenable 57 120 1013 2036 2416 4293 14608 16369 32752 + 848090912 apocalyptic 247 1191 4083 8178 14608 15619 16369 arithmetic 11 57 66 247 302 502 1013 1191 2036 + 9738114 binomial 66 120 brilliant 247 c.decagonal 11 c.square 1013 cake 26 Chen 11 65519 478271 congruent 120 247 302 502 1013 1191 2036 2416 4293 + 2203488 constructible 120 Cunningham 26 120 Curzon 26 1013 131054 45533450 198410786 cyclic 11 247 1013 4083 15619 16369 65519 478271 1048555 4194281 D-number 57 1191 4083 de Polignac 65519 deficient 11 26 57 247 302 502 1013 1191 2036 + 8388584 dig.balanced 11 120 302 502 15619 47840 152637 4537314 10187685 134217700 Duffinian 57 247 1191 1048555 eban 66 2036 economical 11 1013 1191 4293 15619 16369 65519 152637 478271 + 13824739 emirpimes 26 302 502 equidigital 11 1013 1191 4293 15619 16369 65519 152637 478271 + 13824739 evil 57 66 120 1013 1191 2036 4083 8178 47840 + 423281535 factorial 120 Friedman 524268 gapful 120 47840 131054 156190 262125 2203488 10187685 126781020 134217700 + 14875399450 good prime 11 65519 happy 302 2036 32752 1479726 Harshad 120 247 47840 131054 134217700 3464764515 hexagonal 66 120 highly composite 120 hoax 152637 Hogben 57 1191 Honaker 67108837 iban 11 120 247 302 idoneal 57 120 inconsummate 4293 262125 interprime 26 120 4293 47840 8388584 10187685 16777191 Jacobsthal 11 Jordan-Polya 120 junction 1013 2416 88234 262125 13824739 Lehmer 247 Leyland 57 lonely 120 Lucas 11 lucky 152637 magnanimous 11 metadrome 26 57 247 modest 26 2036 Moran 247 nialpdrome 11 66 nude 11 66 oban 11 26 57 66 odious 11 26 247 302 502 2416 4293 14608 15619 + 848090912 palindromic 11 66 palprime 11 pancake 11 2416 panconsummate 11 57 pandigital 11 120 partition 11 pentagonal 247 pernicious 11 26 66 247 302 502 2416 4293 14608 + 8388584 plaindrome 11 26 57 66 247 practical 66 120 14608 32752 47840 2203488 prim.abundant 66 131054 prime 11 1013 15619 16369 65519 478271 13824739 67108837 primeval 1013 Proth 57 pseudoperfect 66 120 8178 14608 32752 47840 131054 524268 repdigit 11 66 repunit 57 1191 self 15619 67108837 848090912 semiprime 26 57 247 302 502 1191 4083 41932745 sliding 11 502 Smith 152637 Sophie Germain 11 1013 sphenic 66 88234 152637 156190 1048555 1310354 4194281 16777191 strobogrammatic 11 strong prime 11 15619 65519 478271 13824739 super Niven 120 super-d 247 15619 16369 65519 156190 2203488 4194281 superabundant 120 tau 524268 2203488 8388584 tetrahedral 120 triangular 66 120 twin 11 65519 478271 uban 11 26 57 66 Ulam 11 26 57 502 1191 2097130 unprimeable 14608 32752 156190 455192 untouchable 120 2036 2416 455192 524268 wasteful 26 57 66 120 247 302 502 2036 2416 + 9738114 weak prime 1013 16369 67108837 Zuckerman 11 Zumkeller 66 120 8178 14608 32752 47840 zygodrome 11 66