[go: up one dir, main page]
Search a number
betrothed pairs
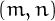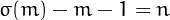Two numbers  $(m,n)$  form a betrothed pair if the sum of nontrivial divisors of one number equals the other, i.e., if  $\sigma(n)-n-1= m$  and  $\sigma(m)-m-1 = n$.

The first numbers which belong to a betrothed pair are (48, 75), (140, 195), (1050, 1925), (1575, 1648), (2024, 2295), (5775, 6128), (8892, 16587), (9504, 20735), (62744, 75495), (186615, 206504) more terms

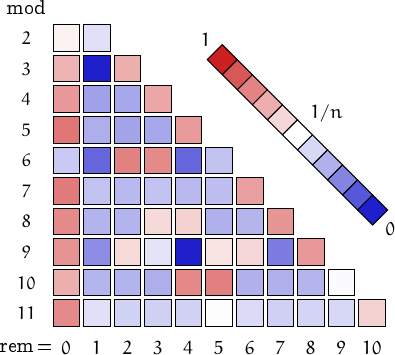
Pictorial representation of remainders (mod 2, 3, ...,11) frequency. For a table of values and more details click here
remainders of betrothed numbers

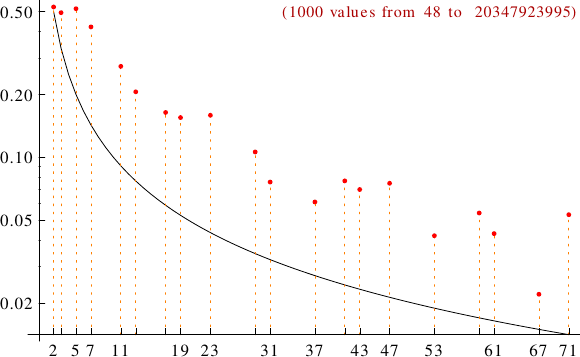
A graph displaying how many betrothed numbers are multiples of the primes p from 2 to 71. In black the ideal line 1/p.
divisibility of betrothed numbers
Useful links Mathworld, Quasiamicable Pair
Wikipedia, Betrothed numbers
OEIS, sequence A005276
Betrothed numbers can also be... (you may click on names or numbers and on + to get more values)

aban 48 75 140 195 1000824 1010000396 2359000280 abundant 48 140 1050 1575 2024 5775 8892 9504 62744 186615 + 41137620 49217084 admirable 140 alternating 1050 16587 amenable 48 140 1648 1925 2024 6128 8892 9504 62744 196664 + 987558704 991087064 apocalyptic 1925 2024 5775 6128 8892 9504 16587 20735 arithmetic 140 195 1050 1925 2024 2295 5775 9504 20735 62744 + 9247095 9345903 binomial 2024 congruent 1575 1648 1925 2295 5775 6128 8892 20735 62744 75495 + 9247095 9345903 constructible 48 Cunningham 48 195 2024 5775 20735 309135 26409320 Curzon 1925 1279950 2576945 7875450 16381925 23939685 175742294 D-number 195 deficient 75 195 1648 1925 2295 6128 16587 20735 75495 206504 + 7890575 9345903 dig.balanced 75 195 8892 16587 62744 587460 2421704 4012184 5644415 9247095 + 178415048 184191111 double fact. 48 Duffinian 75 1575 1925 16587 2576945 evil 48 75 195 1050 1575 1925 2295 5775 6128 9504 + 949977644 953056175 fibodiv 75 Friedman 186615 gapful 140 195 1050 1575 5775 186615 199760 219975 266000 1140020 + 20247751575 20322463175 happy 1575 549219 587460 2576945 3220119 7509159 7890575 harmonic 140 Harshad 48 140 195 1050 2024 9504 62744 266000 312620 573560 + 9456738444 9629379500 highly composite 48 hoax 507759 549219 1057595 2198504 2681019 6618080 12146750 37291625 52389315 66275384 84854315 iban 140 2024 idoneal 48 inconsummate 75 195 16587 206504 219975 266000 309135 544784 549219 interprime 195 1050 1575 2295 9504 2140215 2312024 3676491 4282215 7875450 + 93993830 96751395 Jordan-Polya 48 junction 312620 1348935 1763019 2140215 2226014 2576945 2681019 4311024 6446325 88567059 katadrome 75 lucky 75 195 1575 186615 526575 1459143 2140215 2142945 3010215 3220119 3676491 Lynch-Bell 48 metadrome 48 modest 1648 Moran 195 nialpdrome 75 nonagonal 75 11145066075 nude 48 1575 5775 O'Halloran 140 oban 75 odious 140 1648 2024 8892 196664 219975 526575 573560 817479 1000824 + 987827555 991087064 palindromic 5775 pandigital 75 2573840619 partition 1575 pernicious 48 140 1648 2024 8892 196664 219975 573560 817479 1000824 + 8829792 9247095 plaindrome 48 practical 48 140 1050 2024 8892 9504 62744 196664 199760 266000 + 7875450 8829792 prim.abundant 1575 5775 pseudoperfect 48 140 1050 1575 2024 5775 8892 9504 62744 186615 + 573560 587460 repfigit 75 self 75 16587 196664 544784 1173704 3010215 8713880 23939685 26409320 27862695 + 548544744 987827555 Smith 507759 544784 1057595 1139144 1236536 6446325 7890575 84854315 sphenic 195 507759 super Niven 48 140 1050 super-d 1050 549219 1057595 1524831 1763019 2681019 3010215 3220119 7890575 superabundant 48 tau 8892 9504 199760 266000 2580864 6081680 8829792 14371104 94713300 102019644 + 226964240 313472880 tetrahedral 2024 trimorphic 75 uban 48 75 Ulam 48 1648 16587 196664 1139144 1173704 2142945 2198504 3123735 unprimeable 1648 1925 9504 75495 199760 1057595 1081184 1140020 1233056 1279950 + 8829792 9247095 untouchable 2024 8892 9504 199760 266000 wasteful 48 75 140 195 1050 1575 1648 1925 2024 2295 + 9247095 9345903 Zuckerman 1575 Zumkeller 48 140 1050 1575 2024 5775 8892 9504 62744