repfigit numbers

Let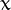

be a number of $n$

digits. Let us define a Fibonacci-like sequence using as seeds the digits of $k$

and then at each step adding the last $n$

terms. If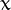

itself appears in the sequence, then it is a repfigit number.

Fibodiv numbers are also named Keith numbers.

For example, 1104 is a repfigit or Keith number because the resulting sequence 1, 1, 0, 4, 6, 11, 21, 42, 80, 154, 297, 573, 1104, contains 1104.

Note that the 6 repfigit numbers with 2 digits are, by definition, fibodiv numbers, too.

The first repfigit numbers are 14, 19, 28, 47, 61, 75, 197, 742, 1104, 1537, 2208, 2580, 3684, 4788, 7385, 7647, 7909, 31331, 34285, 34348, 55604, 62662, 86935, 93993, 120284 more terms

Pictorial representation of remainders (mod 2, 3, ...,11) frequency. For a table of values and more details click here

A graph displaying how many repfigit numbers are multiples of the primes p from 2 to 71. In black the ideal line 1/p.

Useful links Mathworld, Keith Number
Wikipedia, Keith number
OEIS, Sequence A007629

Repfigit numbers can also be... (you may click on names or numbers and on + to get more values)

aban 14 19 28 47 61 75 197 742 abundant 1104 2208 2580 3684 4788 147640 174680 183186 298320 694280 33445755 alt.fact. 19 alternating 14 47 61 amenable 28 61 197 1104 1537 2208 2580 3684 4788 7385 + 925993 7913837 129572008 251133297 apocalyptic 3684 4788 7385 7647 7909 arithmetic 14 19 47 61 197 742 1537 2208 2580 7385 + 355419 925993 1084051 7913837 betrothed 75 binomial 28 brilliant 14 1537 c.decagonal 61 c.heptagonal 197 c.nonagonal 28 c.square 61 c.triangular 19 Carol 47 Catalan 14 Chen 19 47 197 1084051 congruent 14 28 47 61 197 742 1104 2208 2580 4788 + 147640 174680 298320 7913837 Cunningham 28 197 2208 Curzon 14 7385 93993 cyclic 19 47 61 197 1537 7647 7909 31331 34285 86935 925993 1084051 7913837 D-number 7647 d-powerful 62662 decagonal 742 deficient 14 19 47 61 75 197 742 1537 7385 7647 + 355419 925993 1084051 7913837 dig.balanced 19 75 197 742 3684 55604 156146 183186 298320 Duffinian 75 1537 7385 7909 31331 34285 86935 925993 7913837 economical 14 19 47 61 197 1537 34285 129106 1084051 emirp 1084051 emirpimes 7909 7913837 equidigital 14 19 47 61 197 1537 34285 129106 1084051 eRAP 1104 evil 75 197 742 2580 3684 4788 7385 34348 55604 86935 + 7913837 11436171 44121607 129572008 fibodiv 14 19 28 47 61 75 gapful 2580 147640 174680 298320 33445755 happy 19 28 4788 7913837 harmonic 28 Harshad 1104 2208 2580 298320 hex 19 61 hexagonal 28 hoax 34348 86935 hyperperfect 28 iban 14 47 742 1104 idoneal 28 inconsummate 75 31331 interprime 1537 3684 4788 junction 1104 2208 86935 355419 katadrome 61 75 742 Lucas 47 lucky 75 7909 355419 1084051 m-pointer 61 magnanimous 14 47 61 metadrome 14 19 28 47 modest 19 nialpdrome 61 75 742 nonagonal 75 oban 19 28 75 odious 14 19 28 47 61 1104 1537 2208 7647 7909 + 298320 355419 33445755 251133297 pancake 742 panconsummate 14 61 pandigital 19 75 742 perfect 28 pernicious 14 19 28 47 61 1104 1537 2208 7647 120284 298320 355419 Pierpont 19 plaindrome 14 19 28 47 4788 practical 28 1104 2208 2580 4788 174680 298320 694280 prime 19 47 61 197 1084051 Proth 1537 pseudoperfect 28 1104 2208 2580 3684 4788 147640 174680 183186 298320 694280 self 75 1537 3684 7385 7913837 semiprime 14 1537 7647 7909 34285 86935 129106 925993 7913837 44121607 Smith 4788 86935 sphenic 742 7385 31331 156146 strong prime 197 super-d 19 31331 34285 183186 355419 tau 2208 3684 4788 298320 triangular 28 trimorphic 75 truncatable prime 47 197 twin 19 61 197 uban 19 28 47 61 75 Ulam 28 47 197 34285 55604 93993 925993 unprimeable 2580 3684 7385 34348 62662 129106 156146 174680 183186 694280 untouchable 3684 156146 298320 upside-down 19 28 wasteful 28 75 742 1104 2208 2580 3684 4788 7385 7647 + 355419 694280 925993 7913837 weak prime 19 47 61 1084051 Zumkeller 28 1104 2208 2580 3684 4788