Zumkeller numbers

A number

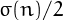is a Zumkeller number if its divisors can be partitioned into two sets with the same sum, which will be $\sigma(n)/2$ .

For example, $12$ is a Zumkeller number because its divisors, i.e., 1, 2, 3, 4, 6, 12, can be partitioned in the two sets {12,2}, and {1,3,4,6} whose common sum is 14.

If $n$ is a Zumkeller number, then $\sigma(n)$ is even and $n$ is perfect or abundant.

All the practical numbers $n$ , with $\sigma(n)$ even are also Zumkeller numbers.

Bhakara Rao & Peng have proved several results on Zumkeller numbers, for example that $n!$ is a Zumkeller numbers for $n\ge 3$ .

The first Zumkeller numbers are 6, 12, 20, 24, 28, 30, 40, 42, 48, 54, 56, 60, 66, 70, 78, 80, 84, 88, 90, 96, 102, 104, 108 more terms

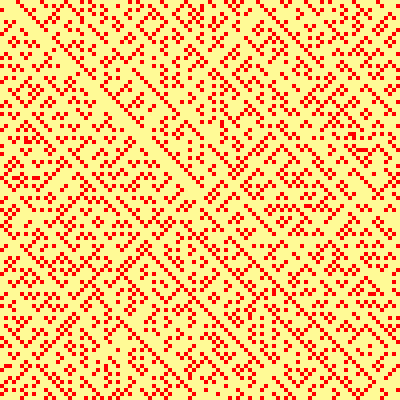
Below, the spiral pattern of Zumkeller numbers up to 10000. See the page on prime numbers for an explanation and links to similar pictures.

Pictorial representation of remainders (mod 2, 3, ...,11) frequency. For a table of values and more details click here

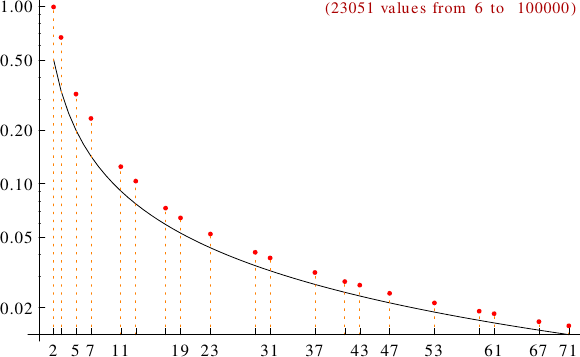
A graph displaying how many Zumkeller numbers are multiples of the primes p from 2 to 71. In black the ideal line 1/p.

Useful links OEIS, Sequence A083207
K.P.S. Bhaskara Rao, Yuejian Peng, On Zumkeller Numbers

Zumkeller numbers can also be... (you may click on names or numbers and on + to get more values)

ABA 24 160 192 + 98304 aban 12 20 24 + 996 abundant 12 20 24 + 100000 Achilles 108 432 500 + 98784 admirable 12 20 24 + 99988 alternating 12 30 54 + 96525 amenable 12 20 24 + 100000 amicable 220 1184 2620 + 79750 apocalyptic 192 220 222 + 30000 arithmetic 20 30 42 + 99996 astonishing 204 216 3078 + 23490 Bell 4140 betrothed 48 140 1050 + 62744 binomial 20 28 56 + 98790 c.heptagonal 736 3256 7568 + 74096 c.nonagonal 28 496 820 + 96580 c.pentagonal 276 456 1266 + 94576 c.triangular 460 760 2584 + 99460 cake 42 176 378 + 95368 Catalan 42 132 1430 + 58786 compositorial 24 192 1728 17280 congruent 20 24 28 + 99996 constructible 12 20 24 + 98688 cube 216 1000 1728 + 85184 Cunningham 24 28 48 + 97968 Curzon 30 54 78 + 99966 D-number 4095 16695 d-powerful 24 132 224 + 99402 decagonal 126 540 1242 + 96876 dig.balanced 12 42 56 + 65280 double fact. 48 384 945 + 46080 droll 240 672 2240 + 93184 Duffinian 1575 14175 39375 + 96075 eban 30 40 42 + 66066 economical 112 160 192 + 100000 enlightened 2176 2560 2744 25000 equidigital 112 160 192 + 99584 eRAP 20 24 1104 + 76640 esthetic 12 54 56 + 89898 Eulerian 66 120 8178 + 47840 evil 12 20 24 + 100000 factorial 24 120 720 + 40320 fibodiv 28 366 3248 + 97494 Fibonacci 2584 46368 Friedman 126 216 736 + 98304 frugal 1280 1536 1792 + 100000 gapful 108 120 132 + 100000 Gilda 78 220 330 + 65676 Giuga 30 858 1722 66198 happy 28 70 176 + 100000 harmonic 28 140 270 + 55860 Harshad 12 20 24 + 100000 heptagonal 112 342 540 + 98704 hexagonal 28 66 120 + 98346 highly composite 12 24 48 + 83160 hoax 84 160 234 + 99860 house 78 1716 5336 + 84540 hungry 82810 hyperperfect 28 496 8128 iban 12 20 24 + 100000 iccanobiF 836 idoneal 12 24 28 + 1848 inconsummate 84 216 272 + 99996 insolite 11112 interprime 12 30 42 + 99990 Jordan-Polya 12 24 48 + 98304 junction 204 208 210 + 99840 Kaprekar 2728 4950 5292 + 82656 katadrome 20 30 40 + 98760 Leyland 54 320 368 + 94932 lonely 120 1340 1344 + 31430 Lucas 5778 lucky 1575 2835 3465 + 97335 Lynch-Bell 12 24 48 + 98136 magic 260 870 6924 + 87836 magnanimous 12 20 30 + 99712 metadrome 12 24 28 + 45678 modest 222 444 618 + 96888 Moran 42 84 114 + 99930 narcissistic 8208 9474 93084 nialpdrome 20 30 40 + 100000 nonagonal 24 204 396 + 98364 nude 12 24 48 + 99936 O'Halloran 12 20 60 + 924 oban 12 20 28 + 996 octagonal 40 96 176 + 99008 odious 28 42 56 + 99988 palindromic 66 88 222 + 89898 pancake 56 352 704 + 97904 panconsummate 12 20 24 + 78 pandigital 78 108 114 + 44790 partition 30 42 56 + 37338 pentagonal 12 70 176 + 98176 perfect 28 496 8128 pernicious 12 20 24 + 99988 Perrin 12 90 486 + 76725 plaindrome 12 24 28 + 78888 power 216 1000 1728 + 100000 powerful 108 216 432 + 100000 practical 12 20 24 + 100000 prim.abundant 12 20 30 + 99988 primeval 10136 primorial 30 210 2310 30030 pronic 12 20 30 + 99540 Proth 7425 49665 pseudoperfect 12 20 24 + 100000 repdigit 66 88 222 + 66666 repfigit 28 1104 2208 + 4788 repunit 40 156 364 + 97656 Rhonda 2835 4752 5664 + 95232 Ruth-Aaron 24 78 104 + 98644 Saint-Exupery 60 480 780 + 97500 Sastry 528 13224 self 20 42 108 + 99980 sliding 20 70 520 + 92500 Smith 378 438 588 + 99920 sphenic 30 42 66 + 99966 straight-line 210 222 234 + 87654 strobogrammatic 88 96 888 + 99066 super Niven 12 20 24 + 100000 super-d 318 336 348 + 99894 superabundant 12 24 48 + 55440 tau 12 24 40 + 99968 taxicab 4104 13832 32832 + 65728 tetrahedral 20 56 84 + 98770 tetranacci 56 108 208 39648 triangular 28 66 78 + 98790 tribonacci 24 504 5768 66012 trimorphic 24 624 90624 uban 12 20 28 + 96 Ulam 28 48 102 + 99774 undulating 252 272 282 + 89898 unprimeable 204 208 320 + 99948 untouchable 88 96 120 + 99988 upside-down 28 258 456 + 81592 vampire 1260 1530 6880 wasteful 12 20 24 + 99996 weird 70 836 4030 + 99890 Woodall 80 15624 Zuckerman 12 24 112 + 93744 zygodrome 66 88 222 + 99988