good primes

good primes

A prime

$p_n$

is said to be good if it $p_n^2

$p_n^2

p_{n-i}\cdot p_{n+i}$"> for every $1\le i<n$ .

For example, $p_5=11$ is a good prime since $11^2=121$ is greater than $7\cdot13=91$ , $5\cdot17=85$ , $3\cdot19=57$ and $2\cdot23=46$ .

Carl Pomerance has proved that, as Selfrigde conjectured, there are infinite good primes.

The earliest runs of 2, 3,..., 7 consecutive good primes start at 37, 557, 1847, 216703, 6929381, 134193727, 15118087477 (this last value found by Jim Fougeron).

The first good primes are 5, 11, 17, 29, 37, 41, 53, 59, 67, 71, 97, 101, 127, 149, 179, 191, 223, 227, 251, 257, 269, 307, 311, 331, 347, 419, 431, 541, 557, 563, 569, 587, 593, 599, 641, 727, 733, 739, 809, 821, 853 more terms

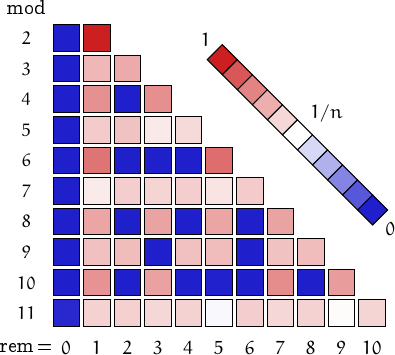
Pictorial representation of remainders (mod 2, 3, ...,11) frequency. For a table of values and more details click here

remainders of good primes

Useful links Wikipedia, Good prime
Carl Pomerance,
The prime number graph, Math. Comp. 33 (1979), 399–408.

Good primes can also be... (you may click on names or numbers and on + to get more values)

a-pointer 11 101 2521 5623 11657 + 198463511 aban 11 17 29 37 41 + 181000679 alt.fact. 101 alternating 29 41 67 101 127 + 189834389 amenable 17 29 37 41 53 + 199968469 apocalyptic 251 541 929 937 1031 + 29983 arithmetic 11 17 29 37 41 + 9993337 balanced p. 53 257 563 593 733 + 199880953 bemirp 10061 10091 19001 c.decagonal 11 101 2531 3251 3511 + 167360051 c.heptagonal 71 2647 50821 54251 139301 + 145857181 c.pentagonal 331 9151 143401 567631 28417531 + 146669851 c.square 41 1861 2521 26681 79601 + 140868113 c.triangular 4621 1173511 1358029 2552581 8904799 + 166368739 Carol 223 Chen 11 17 29 37 41 + 99927257 congruent 29 37 41 53 71 + 9993317 constructible 17 257 65537 Cunningham 17 37 101 127 257 + 139240001 Curzon 29 41 53 593 641 + 199883693 cyclic 11 17 29 37 41 + 9993337 d-powerful 739 2063 2203 2333 3167 + 9887573 de Polignac 127 149 251 331 599 + 99904457 deficient 11 17 29 37 41 + 9993337 dig.balanced 11 37 41 149 541 + 199784461 economical 11 17 29 37 41 + 19988359 emirp 17 37 71 97 149 + 199968443 equidigital 11 17 29 37 41 + 19988359 esthetic 67 101 Eulerian 11 65519 evil 17 29 53 71 101 + 199968443 fibodiv 149 Fibonacci 1597 Friedman 127 347 16879 48751 48757 + 937571 Gilda 29 happy 97 331 563 739 937 + 9993337 hex 37 127 331 4447 13669 + 169989769 Hogben 307 3907 6007 110557 288907 + 196770757 Honaker 3433 4903 6563 6653 7411 + 199022107 hungry 17 iban 11 17 41 71 101 + 747401 idoneal 37 inconsummate 431 563 821 1871 2609 + 999953 Jacobsthal 11 junction 101 307 311 1009 1213 + 99904439 katadrome 41 53 71 97 431 + 8521 Kynea 1087 263167 Leyland 17 593 lonely 53 Lucas 11 29 lucky 37 67 127 223 307 + 9993223 m-pointer 61211 312161 magnanimous 11 29 41 67 101 + 602081 metadrome 17 29 37 59 67 + 1245689 modest 29 59 311 599 733 + 172001287 Motzkin 127 nialpdrome 11 41 53 71 97 + 99754411 nude 11 oban 11 17 29 37 53 + 967 odious 11 37 41 59 67 + 199968539 Ormiston 40693 94397 112997 131413 131431 + 199882217 palindromic 11 101 191 727 929 + 195353591 palprime 11 101 191 727 929 + 195353591 pancake 11 29 37 67 191 + 186331861 panconsummate 11 37 53 59 127 + 331 pandigital 11 partition 11 101 pernicious 11 17 37 41 59 + 9993337 Perrin 17 29 853 Pierpont 17 37 97 257 3457 65537 plaindrome 11 17 29 37 59 + 133333777 prime 11 17 29 37 41 + 199968539 primeval 37 1367 13679 Proth 17 41 97 257 641 + 190857217 repdigit 11 repunit 127 307 3907 6007 110557 + 196770757 self 53 97 569 727 929 + 199883539 self-describing 12103331 sliding 11 29 101 641 Sophie Germain 11 29 41 53 179 + 199883693 star 37 541 937 2521 46993 + 114607621 strobogrammatic 11 101 119611 strong prime 11 17 29 37 41 + 99927257 super-d 127 269 331 419 431 + 9993019 tetranacci 29 tribonacci 149 trimorphic 251 truncatable prime 17 29 37 53 59 + 99537547 twin 11 17 29 41 59 + 199968539 uban 11 17 29 37 41 + 93000097 Ulam 11 53 97 431 739 + 9992681 undulating 101 191 727 929 upside-down 37 9371 1245689 33682477 91864291 98773321 weakly prime 5564453 59160317 61589579 126517543 Wieferich 3511 Woodall 17 191 Zuckerman 11 zygodrome 11 11777 33311 2244499 117770011