[go: up one dir, main page]
Search a number
repunits
A number  $n$  is a repunit in a base  $b$  it all the digits in its representation in base  $b$  are equal to 1.

For example, 1, 1111, and 111111 are repunits in base 10, while  $(1111)_7=400$  is a repunit in base 7.

In general, a repunit  $R_b(n)$  in base  $b1$">  made of  $n$  repetitions of the digit  $1$ has value

\[ R_b(n) = \frac{b^n-1}{b-1}\,. \]

The repdigits composed by repeated ones are called repunits.

Since every number  $n2$">  can be represented as  $11$  in base  $n-1$, I consider only nontrivial repunits, i.e., those that contains at least 3 ones in some base.

The first nontrivial repunits are , 13, 15, 21, 31, 40, 43, 57, 63, 73, 85, 91, 111, 121, 127, 133, 156, 157, 183, 211, 241, 255, 259, 273, 307, 341, 343, 364, 381, 400, 421more terms

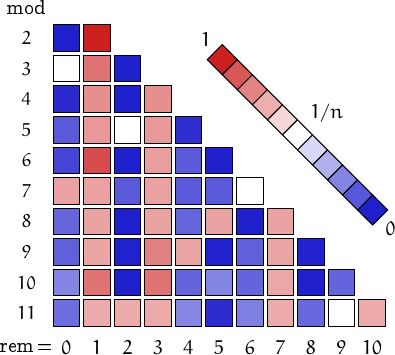
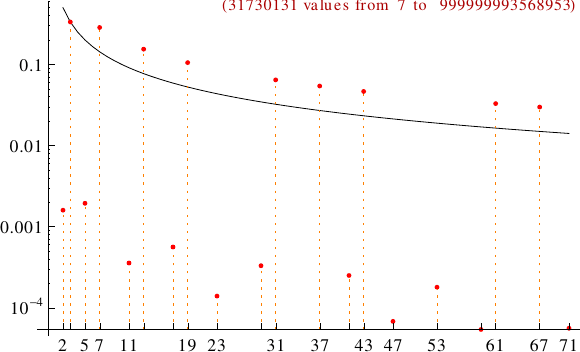
Pictorial representation of remainders (mod 2, 3, ...,11) frequency. For a table of values and more details click here
remainders of repunits

A graph displaying how many repunits are multiples of the primes p from 2 to 71. In black the ideal line 1/p.
divisibility of repunits
Useful links Mathworld, Repunit
Wikipedia, Repunit
OEIS, Sequence A053696
Repunits can also be... (you may click on names or numbers and on + to get more values)

a-pointer 13 24181 121453 + 9487636621 aban 13 15 21 + 999999000001 abundant 40 156 364 + 49565920 Achilles 7906143973 admirable 40 364 4095 alternating 21 43 63 + 981036363 amenable 13 21 40 + 999856021 apocalyptic 157 820 871 + 29757 arithmetic 13 15 21 + 9995083 astonishing 15 balanced p. 157 211 1123 + 9945973171 Bell 15 binomial 15 21 91 + 4734390674091 brilliant 15 21 121 + 999413383 c.decagonal 31 211 781 + 729500152756861 c.heptagonal 43 463 5461 + 300797044280503 c.nonagonal 91 703 820 + 231627523606480 c.octagonal 121 c.pentagonal 31 601 43891 + 131602780302451 c.square 13 85 421 + 745519146510613 c.triangular 31 85 2971 + 26835295698121 cake 15 Carmichael 5310721 2278677961 9593125081 29859667201 Chen 13 31 127 + 99790111 congruent 13 15 21 + 9969807 constructible 15 40 85 + 4294967295 cube 343 Cunningham 15 31 63 + 562949953421311 Curzon 21 273 341 + 199953741 cyclic 13 15 31 + 9982441 D-number 15 21 57 + 6993381 d-powerful 43 63 463 + 9573285 de Polignac 127 757 1807 + 99930013 decagonal 85 2047 1307020 deceptive 91 259 703 + 30244862011 deficient 13 15 21 + 9995083 dig.balanced 15 21 156 + 199812361 double fact. 15 Duffinian 21 57 63 + 9988761 eban 40 52060 economical 13 15 21 + 19994313 emirp 13 31 73 + 199162657 emirpimes 15 85 183 + 99810091 equidigital 13 15 21 + 19994313 eRAP 21058218111 esthetic 21 43 121 + 101010101010101 Eulerian 57 1191 evil 15 40 43 + 999792781 fibodiv 183 87321 Fibonacci 13 21 Friedman 121 127 343 + 597871 frugal 343 105301 179353 + 891589741 gapful 121 341 400 + 99992242441 Gilda 364 good prime 127 307 3907 + 196770757 happy 13 31 91 + 9988761 Harshad 21 40 63 + 9988303423 heptagonal 6175 18361 27931 + 29093062258603 hex 91 127 1261 + 346332937450507 hexagonal 15 91 703 + 160829915470003 hoax 85 364 1111 + 99351057 Hogben 13 21 31 + 999999993568953 Honaker 5701 8011 98911 + 975906361 hyperperfect 21 1333 16513 + 1951956868501 iban 21 40 43 + 777043 iccanobiF 13 idoneal 13 15 21 + 1365 inconsummate 63 381 553 + 995007 insolite 111 111111111 interprime 15 21 111 + 99450757 Jacobsthal 21 43 85 + 375299968947541 junction 111 307 507 + 99870043 Kaprekar 703 1111111111 katadrome 21 31 40 + 87321 Lehmer 15 85 91 + 984588837961 Leyland 57 lonely 211 lucky 13 15 21 + 9976123 Lynch-Bell 15 m-pointer 1123 magic 15 111 magnanimous 21 43 85 + 6007 metadrome 13 15 57 + 259 modest 13 111 133 + 1111111111 Moran 21 63 111 + 99390931 Motzkin 21 127 nialpdrome 21 31 40 + 111111111111111 nonagonal 111 651 99541 + 423236211589731 nude 15 111 1111 + 118612344 O'Halloran 156 oban 13 15 57 + 993 octagonal 21 40 133 + 375299968947541 odious 13 21 31 + 999982507 Ormiston 37831 530713 7681213 + 1997598331 palindromic 111 121 343 + 755971313179557 palprime 757 30103 pancake 121 211 781 + 372759573255307 panconsummate 15 21 31 + 1093 pandigital 15 21 156 + 15401701 partition 15 pentagonal 651 2380 5551 + 576009576039801 pernicious 13 21 31 + 9988761 persistent 10158926473 10865247933 12859673401 + 79865303421 Pierpont 13 73 plaindrome 13 15 57 + 111111111111111 Poulet 341 2047 4033 + 421925923038061 power 121 343 400 powerful 121 343 400 7906143973 practical 40 156 364 + 9984816 prim.abundant 364 4095 21562515 prime 13 31 43 + 999999000001 primeval 13 pronic 156 3906 97656 + 596046447753906 Proth 13 57 241 + 274877382657 pseudoperfect 40 156 364 + 980200 repdigit 111 1111 11111 + 111111111111111 Ruth-Aaron 15 154843 1236600 + 19369541451 Saint-Exupery 13709280 Sastry 183 40495 self 31 121 211 + 999160491 semiprime 15 21 57 + 99910021 sliding 133 Smith 85 121 1111 + 99351057 Sophie Germain 28792661 78914411 943280801 7294932341 sphenic 255 273 651 + 99950007 square 121 400 star 13 73 121 + 308836698141973 straight-line 111 1111 11111 + 111111111111111 strobogrammatic 111 1111 10101 + 111111111111111 strong prime 127 307 757 + 99211561 super Niven 40 400 super-d 31 127 381 + 9976123 tau 40 156 3280 + 943531280 taxicab 2436429101743 12166921836433 tetrahedral 364 tetranacci 15 triangular 15 21 91 + 231627523606480 tribonacci 13 truncatable prime 13 31 43 + 5113 twin 13 31 43 + 998907631 uban 13 15 21 + 96040049000007 Ulam 13 57 241 + 9894171 undulating 121 343 585 + 101010101010101 unprimeable 1464 3280 69905 + 9846355 untouchable 20440 60880 621436 + 866496 upside-down 73 91 41182996 98392919181721 wasteful 40 57 63 + 9995083 weak prime 13 31 43 + 99990001 weakly prime 23402421463 Wieferich 1093 22953 Woodall 63 1023 2047 + 137438953471 Zeisel 1885 Zuckerman 15 111 1111 + 1111111111 Zumkeller 40 156 364 + 97656 zygodrome 111 1111 11111 + 111111111111111