[go: up one dir, main page]
Search a number
Wieferich numbers
A number  $n$  is a Wieferich number if it holds  $2^{\phi(n)}\equiv 1 \pmod {n^2}$.

The formula above, if  $n$  is a prime, is equivalent to  $2^{n-1}\equiv 1 \pmod {n^2}$, however only two such numbers, called Wieferich primes are known, namely 1093 and 3511.

The first Wieferich numbers are 1093, 3279, 3511, 7651, 10533, 14209, 17555, 22953, 31599, 42627, 45643, 52665, 68859 more terms

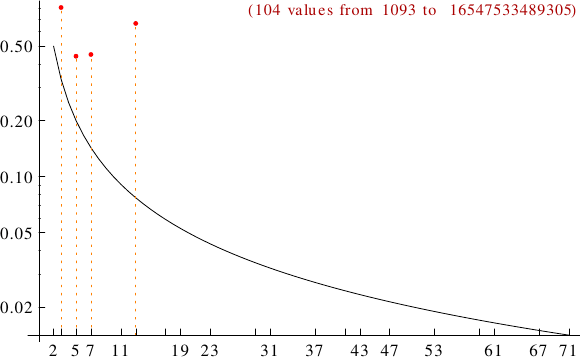
Currently 104 Wieferich numbers are known, the largest being 16547533489305. T. Agoh, K. Dilcher & L. Skula have proved that a larger Wieferich number can exist only if other Wieferich primes exist, apart the 1093 and 3511.

Similarly, two primes  $p$  and  $q$  such that  $p^{q-1}\equiv 1 \pmod {q^2}$  and  $q^{p-1}\equiv 1 \pmod {p^2}$, are called a Wieferich pair.

Only 7 such pairs are known : (2, 1093), (3, 1006003), (5, 1645333507), (5, 188748146801), (83, 4871), (911, 318917), and (2903, 18787).

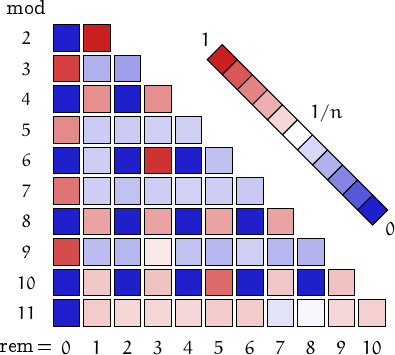
Pictorial representation of remainders (mod 2, 3, ...,11) frequency. For a table of values and more details click here
remainders of Wieferich numbers

A graph displaying how many Wieferich numbers are multiples of the primes p from 2 to 71. In black the ideal line 1/p.
divisibility of Wieferich numbers
Useful links Wikipedia, Wieferich prime
Mathworld, Wieferich Prime
OEIS, Sequence A077816
Wieferich numbers can also be... (you may click on names or numbers and on + to get more values)

alternating 298389 410787 1232361 amenable 1093 10533 14209 22953 52665 94797 127881 136929 298389 473985 + 241763949 349214593 518065605 748316985 apocalyptic 3279 3511 7651 10533 14209 17555 22953 arithmetic 1093 3279 3511 7651 10533 14209 17555 22953 42627 45643 + 2053935 2685501 3837523 6161805 binomial 6161805 brilliant 3837523 c.decagonal 3511 Chen 3511 congruent 1093 3279 3511 10533 14209 31599 94797 99463 127881 228215 + 895167 2053935 2685501 6161805 Curzon 10533 52665 127881 298389 6161805 cyclic 1093 3511 3837523 D-number 3279 10533 d-powerful 22953 de Polignac 3837523 deficient 1093 3279 3511 7651 10533 14209 17555 22953 31599 42627 + 2685501 3697083 3837523 6161805 dig.balanced 14209 45643 157995 298389 684645 Duffinian 3279 7651 10533 14209 17555 22953 31599 42627 45643 68859 + 228215 1232361 3697083 3837523 economical 1093 3511 10533 17555 emirp 3511 emirpimes 17555 45643 equidigital 1093 3511 10533 17555 evil 1093 3279 10533 17555 22953 31599 45643 52665 68859 127881 + 349214593 402939915 648541387 725291847 gapful 157995 473985 684645 2685501 6161805 18485415 172688535 1208819745 1346970573 1554196815 + 32638133115 47143970055 60613675785 68096845635 good prime 3511 happy 1093 10533 42627 157995 684645 Harshad 22953 298389 2685501 6161805 172688535 1208819745 1554196815 1945624161 2244950955 3626459235 hex 7651 hoax 2685501 Hogben 22953 inconsummate 94797 127881 473985 interprime 157995 684645 18485415 junction 14209 136929 2053935 57562845 katadrome 7651 lucky 1093 10533 14209 22953 94797 2053935 3697083 Moran 22953 nialpdrome 7651 odious 3511 7651 14209 42627 94797 99463 157995 298389 895167 2053935 + 249438995 448990191 518065605 748316985 panconsummate 1093 pandigital 298389 pernicious 14209 42627 99463 895167 2685501 3697083 6161805 prime 1093 3511 Proth 14209 repunit 1093 22953 self 17555 410787 18485415 26862661 518065605 semiprime 3279 7651 10533 14209 17555 45643 3837523 sphenic 22953 42627 52665 99463 136929 228215 11512569 19187615 26862661 49887799 star 1093 strong prime 3511 super-d 10533 42627 127881 triangular 6161805 twin 1093 Ulam 52665 94797 473985 unprimeable 6161805 wasteful 3279 7651 14209 22953 31599 42627 45643 52665 68859 94797 + 2685501 3697083 3837523 6161805 weak prime 1093