droll numbers

droll numbers

Mario Velucchi called a number 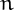$n$

$n$

droll if the sum of its even prime factors equals the sum of its odd prime factors.

For example, $718848=2^{11}\cdot3^3\cdot13$ is a droll number because $2\cdot11 = 3\cdot3 + 13$ .

Up to $10^{18}$ there are only 5902 droll numbers.

The first droll numbers are 72, 240, 672, 800, 2240, 4224, 5184, 6272, 9984, 14080, 17280, 33280, 39424, 48384 more terms

Pictorial representation of remainders (mod 2, 3, ...,11) frequency. For a table of values and more details click here

remainders of droll numbers

A graph displaying how many droll numbers are multiples of the primes p from 2 to 71. In black the ideal line 1/p.

divisibility of droll numbers

Useful links OEIS, Sequence A019507

Droll numbers can also be... (you may click on names or numbers and on + to get more values)

ABA 72 800 5184 6272 192000 + 6946606284800 8173092077568 9748267139072 aban 72 240 672 800 512000000 + 122880000000 344064000000 409600000000 abundant 72 240 672 800 2240 + 41779200 42172416 46080000 Achilles 72 800 6272 247808 1384448 + 29491200000000 37205456191488 49127043891200 admirable 672 alternating 72 672 12189696 78381056 270729216 amenable 72 240 672 800 2240 + 916455424 930349056 995328000 apocalyptic 800 2240 5184 6272 9984 14080 17280 arithmetic 672 14080 116736 1792000 2396160 + 5275648 6709248 7987200 compositorial 17280 congruent 240 9984 14080 17280 39424 + 7241728 7987200 9461760 constructible 240 52224 174080 cube 373248 13824000 303464448 512000000 11239424000 + 142432926695424 191102976000000 809140700053504 Cunningham 4224 d-powerful 2240 4224 39424 dig.balanced 240 247808 Duffinian 800 5184 6272 57600 247808 640000 1384448 5017600 economical 2240 5184 6272 14080 17280 + 16187392 17842176 19005440 equidigital 2240 5184 6272 14080 17280 + 8404992 12533760 17842176 evil 72 240 4224 9984 17280 + 902430720 916455424 995328000 Friedman 39424 93184 373248 389120 487424 565248 frugal 192000 373248 640000 1089536 1244160 + 916455424 930349056 995328000 gapful 240 800 2240 4224 5184 + 91163197440 94466211840 98524200960 happy 33280 174080 192000 304128 2396160 + 5275648 8404992 9461760 harmonic 672 Harshad 72 240 800 2240 4224 + 9289728000 9751756800 9767485440 highly composite 240 iban 72 240 2240 4224 idoneal 72 240 inconsummate 9984 451584 537600 718848 interprime 72 240 4224 5184 116736 + 46080000 59473920 78446592 Jordan-Polya 72 240 5184 17280 57600 + 535088332800000 722204136308736 847579919155200 junction 39424 2838528 33161216 35094528 40632320 53215232 katadrome 72 Lynch-Bell 672 nialpdrome 72 800 9984 640000 nude 672 4224 48384 487424 3483648 4214784 oban 800 odious 672 800 2240 5184 6272 + 871628800 877658112 930349056 palindromic 4224 48384 panconsummate 72 pernicious 72 672 800 2240 4224 + 5275648 5701632 8404992 power 5184 57600 373248 451584 640000 + 36859543552000 45014008725504 46902116614144 powerful 72 800 5184 6272 57600 + 873777843929088 949670956236800 994721136640000 practical 72 240 672 800 2240 + 7987200 8404992 9461760 pronic 72 240 pseudoperfect 72 240 672 800 2240 + 585728 640000 718848 Saint-Exupery 260717936640 494130954240 6801567252480 251909898240000 self 6272 14080 304128 7241728 17842176 + 468582400 521404416 732168192 Smith 37879808 square 5184 57600 451584 640000 5017600 + 722204136308736 784932116889600 994721136640000 super Niven 240 800 super-d 33280 48384 161280 247808 1792000 3379200 6848512 superabundant 240 tau 72 240 672 2240 4224 + 836075520 902430720 995328000 taxicab 34780741632 783210774528 1288175616000 28278031122432 29007806464000 636779367497728 uban 72 Ulam 72 800 5184 14080 48384 + 565248 3760128 9461760 unprimeable 9984 17280 39424 174080 247808 + 6848512 7987200 9461760 untouchable 4224 5184 33280 161280 373248 451584 wasteful 72 240 672 800 4224 + 6709248 7987200 9461760 Zuckerman 672 4224 487424 3483648 4214784 Zumkeller 240 672 2240 4224 9984 + 48384 52224 93184