Jacobsthal numbers

Jacobsthal numbers are defined by the recurrence 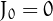$J_0=0$

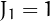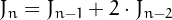and $J_n=J_{n-1}+2\cdot J_{n-2}$ for

1$">.

Their closed form is

$J_n = \frac{2^n-(-1)^n}{3}\,.$

$J_n$ has several combinatorial interpretations. For example, it is equal to the number of ways a rectangle $(n-1)\times 2$ can by tiles using dominoes and $2\times 2$ squares.

Two infinite sums (the first for $$\alpha$ 2$">)

$\sum_{n=1}^{\infty}\frac{J_n}{\alpha^n}=\frac{\alpha}{(\alpha+1)(\alpha-2)}\,\quad\quad \sum_{n=1}^{\infty}\frac{J_n}{n!} = \frac{e^3-1}{3e}\,.$

The first Jacobsthal numbers are 1, 3, 5, 11, 21, 43, 85, 171, 341, 683, 1365, 2731, 5461, 10923, 21845, 43691, 87381, 174763, 349525 more terms

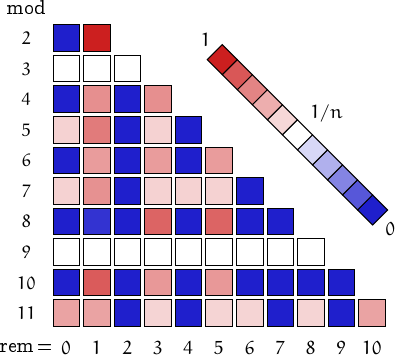
Pictorial representation of remainders (mod 2, 3, ...,11) frequency. For a table of values and more details click here

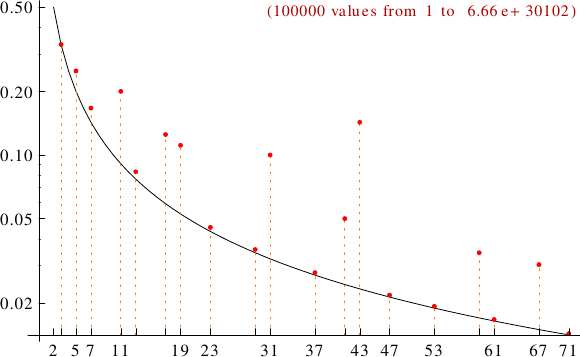
A graph displaying how many Jacobsthal numbers are multiples of the primes p from 2 to 71. In black the ideal line 1/p.

Useful links Mathworld, Jacobsthal Number
Wikipedia, Jacobsthal number
OEIS, Sequence A001045

Jacobsthal numbers can also be... (you may click on names or numbers and on + to get more values)

a-pointer 11 aban 11 21 43 85 171 341 683 abundant 5592405 alternating 21 43 85 341 10923 amenable 21 85 341 1365 5461 21845 87381 349525 1398101 5592405 22369621 89478485 357913941 apocalyptic 10923 21845 arithmetic 11 21 43 85 341 683 1365 2731 5461 10923 + 699051 1398101 2796203 5592405 binomial 21 171 1365 brilliant 21 341 22369621 c.decagonal 11 c.heptagonal 43 5461 c.square 85 c.triangular 85 Chen 11 683 2731 43691 congruent 21 85 341 1365 5461 21845 87381 349525 1398101 5592405 constructible 85 21845 1431655765 Curzon 21 341 1365 21845 87381 1398101 89478485 cyclic 11 43 85 341 683 2731 5461 21845 43691 174763 1398101 2796203 D-number 21 d-powerful 43 2796203 decagonal 85 deceptive 5461 1398101 22369621 deficient 11 21 43 85 171 341 683 1365 2731 5461 + 349525 699051 1398101 2796203 dig.balanced 11 21 699051 Duffinian 21 85 171 341 5461 21845 87381 699051 1398101 economical 11 21 43 683 2731 43691 174763 1398101 2796203 emirpimes 85 341 equidigital 11 21 43 683 2731 43691 174763 1398101 2796203 esthetic 21 43 Eulerian 11 evil 43 85 683 1365 10923 21845 174763 349525 2796203 5592405 44739243 89478485 715827883 Fibonacci 21 Friedman 21845 43691 87381 gapful 341 1365 11184811 357913941 Gilda 683 good prime 11 happy 683 5592405 Harshad 21 171 1365 1398101 hoax 85 Hogben 21 43 hyperperfect 21 iban 11 21 43 171 341 idoneal 21 85 1365 inconsummate 87381 interprime 21 10923 junction 349525 44739243 katadrome 21 43 85 Lehmer 85 5461 21845 1398101 11184811 22369621 1431655765 91625968981 Lucas 11 lucky 21 43 171 1365 10923 699051 magnanimous 11 21 43 85 683 Moran 21 171 Motzkin 21 nialpdrome 11 21 43 85 nude 11 oban 11 85 683 octagonal 21 341 5461 87381 1398101 22369621 357913941 5726623061 91625968981 1466015503701 23456248059221 375299968947541 odious 11 21 171 341 2731 5461 43691 87381 699051 1398101 11184811 22369621 178956971 357913941 palindromic 11 171 palprime 11 pancake 11 5461 panconsummate 11 21 43 85 pandigital 11 21 699051 partition 11 pernicious 11 21 171 341 2731 5461 699051 1398101 plaindrome 11 Poulet 341 5461 1398101 22369621 178956971 5726623061 45812984491 91625968981 733007751851 23456248059221 46912496118443 prime 11 43 683 2731 43691 174763 2796203 715827883 repdigit 11 repunit 21 43 85 341 1365 5461 21845 87381 349525 1398101 + 5864062014805 23456248059221 93824992236885 375299968947541 semiprime 21 85 341 5461 22369621 sliding 11 Smith 85 Sophie Germain 11 683 43691 2796203 sphenic 10923 21845 699051 1398101 11184811 strobogrammatic 11 strong prime 11 43691 2796203 super-d 2731 87381 triangular 21 171 truncatable prime 43 683 twin 11 43 2731 174763 715827883 uban 11 21 43 85 Ulam 11 341 87381 699051 undulating 171 wasteful 85 171 341 1365 5461 10923 21845 87381 349525 699051 5592405 weak prime 43 683 2731 174763 Zuckerman 11 zygodrome 11