deficient numbers

A number

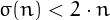is said to be deficient if $\sigma(n) < 2\cdot n$ , i.e., if the sum of the proper divisors of $n$

is smaller than $n$

For example, 7 is deficient, since its only proper divisor is 1, which is smaller than 7. It is easy to see that all the primes, their powers and all the semiprimes apart 6, are deficient.

Moreover, if a number is perfect or deficient, then all its proper divisors are deficient.

J.Sándor has proved that for $n$ sufficiently large there is always a deficient number between $n$ and $n+(\log n)^2$ .

About 3/4 of the integers are deficient. The first deficient numbers are 1, 2, 3, 4, 5, 7, 8, 9, 10, 11, 13, 14, 15, 16, 17, 19, 21, 22 more terms

Below, the spiral pattern of deficient numbers up to 2500. See the page on prime numbers for an explanation and links to similar pictures.

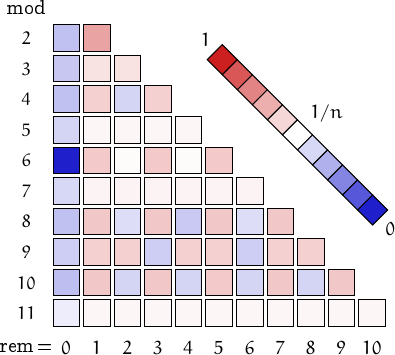
Pictorial representation of remainders (mod 2, 3, ...,11) frequency. For a table of values and more details click here

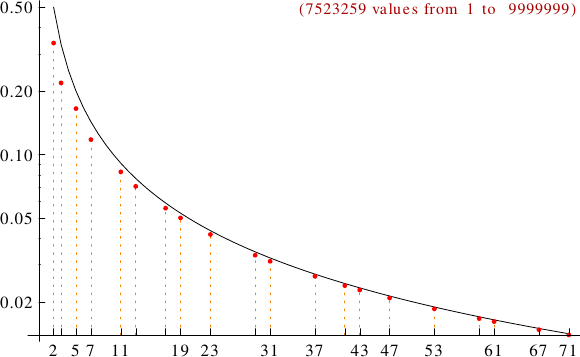
A graph displaying how many deficient numbers are multiples of the primes p from 2 to 71. In black the ideal line 1/p.

Useful links Mathworld, Deficient Number
Wikipedia, Deficient number
OEIS, Sequence A005100

Deficient numbers can also be... (you may click on names or numbers and on + to get more values)

a-pointer 11 13 101 + 9999347 ABA 32 50 64 + 9981512 aban 10 11 13 + 9000999 Achilles 675 1125 1323 + 9981512 alt.fact. 19 101 619 + 3301819 alternating 10 14 16 + 9898989 amenable 13 16 17 + 9999997 amicable 284 1210 2924 + 9892936 anti-perfect 244 285 133857 apocalyptic 157 218 226 + 29999 arithmetic 11 13 14 + 9999999 astonishing 15 27 429 + 7103835 automorphic 25 76 376 + 2890625 balanced p. 53 157 173 + 9999937 Bell 15 52 203 + 4213597 bemirp 1061 1091 1601 + 1909081 betrothed 75 195 1648 + 9345903 binomial 10 15 21 + 9992685 brilliant 10 14 15 + 9999811 c.decagonal 11 31 61 + 9989911 c.heptagonal 22 43 71 + 9990436 c.nonagonal 10 55 91 + 9983746 c.octagonal 25 49 81 + 9991921 c.pentagonal 16 31 51 + 9995001 c.square 13 25 41 + 9994921 c.triangular 10 19 31 + 9996214 cake 15 26 64 + 9886826 Canada 125 581 8549 16999 Carmichael 561 1105 1729 + 9890881 Carol 47 223 959 + 4190207 Catalan 14 429 4862 Chen 11 13 17 + 9999991 congruent 13 14 15 + 9999999 constructible 10 15 16 + 8913032 cube 27 64 125 + 9938375 Cullen 25 65 161 + 9961473 Cunningham 10 15 17 + 9999999 Curzon 14 21 26 + 9999981 cyclic 11 13 15 + 9999997 D-number 15 21 33 + 7043133 d-powerful 43 63 89 + 9999865 de Polignac 127 149 251 + 9999997 decagonal 10 27 52 + 9993501 deceptive 91 259 451 + 9989161 dig.balanced 10 11 15 + 9999994 double fact. 15 105 Duffinian 16 21 25 + 9999997 eban 32 34 44 + 6066064 economical 10 11 13 + 9999991 emirp 13 17 31 + 9999971 emirpimes 15 26 39 + 9999998 enlightened 250 256 2048 + 5117695 equidigital 10 11 13 + 9999991 eRAP 98 170 1274 + 9960047 esthetic 10 21 23 + 9898989 Eulerian 11 26 57 + 8388584 evil 10 15 17 + 9999999 fibodiv 14 19 47 + 9869390 Fibonacci 13 21 34 + 9227465 Friedman 25 121 125 + 999163 frugal 125 128 243 + 9999803 gapful 105 110 121 + 9999985 Gilda 29 49 110 + 4848955 good prime 11 17 29 + 9993337 happy 10 13 19 + 9999992 Harshad 10 21 27 + 9999950 heptagonal 34 55 81 + 9987004 hex 19 37 61 + 9997351 hexagonal 15 45 91 + 9988215 hoax 22 58 85 + 9999922 Hogben 13 21 31 + 9995083 Honaker 131 263 457 + 9987001 house 32 155 271 + 9716393 hungry 17 74 2003 + 7339199 hyperperfect 21 301 325 + 9699181 iban 10 11 14 + 777777 iccanobiF 13 39 124 + 9679838 idoneal 10 13 15 + 1365 impolite 16 32 64 + 8388608 inconsummate 62 63 65 + 999995 insolite 111 interprime 15 21 26 + 9999982 Jacobsthal 11 21 43 + 2796203 Jordan-Polya 16 32 64 + 8388608 junction 101 103 105 + 9999961 Kaprekar 45 55 99 + 9999999 katadrome 10 21 31 + 9876543 Kynea 23 79 287 + 4198399 Lehmer 15 51 85 + 9997351 Leyland 17 32 57 + 9865625 lonely 23 53 211 + 4652429 Lucas 11 29 47 + 7881196 lucky 13 15 21 + 9999997 Lynch-Bell 15 124 128 + 9315 m-pointer 23 61 1123 + 9111341 magic 15 34 65 + 9951391 magnanimous 11 14 16 + 9959374 metadrome 13 14 15 + 3456789 modest 13 19 23 + 9999999 Moran 21 27 45 + 9999950 Motzkin 21 51 127 + 2356779 narcissistic 153 370 371 + 9926315 nialpdrome 10 11 21 + 9999999 nonagonal 46 75 111 + 9992125 nude 11 15 22 + 9999999 O'Halloran 44 116 oban 10 11 13 + 999 octagonal 21 65 133 + 9988225 odious 11 13 14 + 9999998 Ormiston 1913 1931 18379 + 9994631 palindromic 11 22 33 + 9999999 palprime 11 101 131 + 9989899 pancake 11 16 22 + 9997157 panconsummate 10 11 14 + 3097 pandigital 11 15 19 + 9998163 partition 11 15 22 + 9289091 pentagonal 22 35 51 + 9998795 pernicious 10 11 13 + 9999998 Perrin 10 17 22 + 9141872 Pierpont 13 17 19 + 8503057 plaindrome 11 13 14 + 9999999 Poulet 341 561 645 + 9995671 power 16 25 27 + 9991921 powerful 16 25 27 + 9991921 practical 16 32 64 + 8388608 prime 11 13 17 + 9999991 primeval 13 37 107 + 1234679 pronic 110 182 506 + 9969806 Proth 13 17 25 + 9998337 rare 65 621770 repdigit 11 22 33 + 9999999 repfigit 14 19 47 + 7913837 repunit 13 15 21 + 9995083 Rhonda 5265 5439 12985 + 9892552 Ruth-Aaron 15 16 25 + 9997417 Sastry 183 328 715 + 2066115 self 31 53 64 + 9999998 self-describing 22 4444 224444 444422 semiprime 10 14 15 + 9999998 sliding 11 25 29 + 9868025 Smith 22 27 58 + 9999922 Sophie Germain 11 23 29 + 9999653 sphenic 105 110 130 + 9999994 square 16 25 49 + 9991921 star 13 37 73 + 9992341 straight-line 111 123 135 + 9999999 strobogrammatic 11 69 101 + 9998666 strong prime 11 17 29 + 9999971 subfactorial 44 265 14833 1334961 super Niven 10 50 110 + 1000010 super-d 19 31 69 + 9999981 tau 128 136 152 + 9999992 taxicab 1729 20683 40033 + 9443761 tetrahedral 10 35 165 + 9886435 tetranacci 15 29 401 + 7555935 triangular 10 15 21 + 9992685 tribonacci 13 44 81 + 4700770 trimorphic 25 49 51 + 9999999 truncatable prime 13 17 23 + 9986113 twin 11 13 17 + 9999973 uban 10 11 13 + 9000099 Ulam 11 13 16 + 9999999 undulating 101 121 131 + 9898989 unprimeable 206 322 325 + 9999988 untouchable 52 124 146 + 999970 upside-down 19 37 46 + 9995111 vampire 1395 1435 1827 + 939658 wasteful 22 26 33 + 9999999 weak prime 13 19 23 + 9999991 weakly prime 294001 505447 584141 + 9931447 Wieferich 1093 3279 3511 + 6161805 Woodall 17 23 63 + 9961471 Zeisel 105 1419 1729 + 9773731 Zuckerman 11 15 111 + 9939915 zygodrome 11 22 33 + 9999999