A number is undulating in base 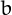 if it has at least 3 digits and it is made of exactly two distict digits which alternate, like 252 or 373737 in base 10 or 21=(10101)2.
if it has at least 3 digits and it is made of exactly two distict digits which alternate, like 252 or 373737 in base 10 or 21=(10101)2.
David Moews has proved that there are only 4 undulating squares, namely 121, 484, 676, and 69696.
The first numbers which are undulating in at least 2 bases b <= 16 are 10, 46, 50, 55, 67, 78, 85, 92, 98, 100, 104, 109, 119, 121, 130, 135, 136, 141, 145, 151, 154, 164, 166, 170, 178, 181, 182, 185, 191, 197, 200
Below, the spiral pattern of undulating numbers up to . See the page on prime numbers for an explanation and links to similar pictures.
The first undulating numbers in base 10 are 101, 121, 131, 141, 151, 161, 171, 181, 191, 202, 212, 232, 242, 252, 262, 272, 282, 292, 303, 313 more terms
Pictorial representation of remainders (mod 2, 3, ...,11) frequency. For a table of values and more details click here

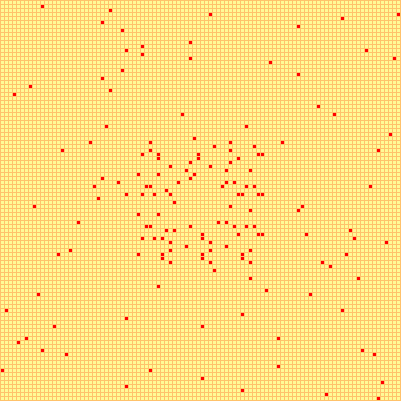
A graph displaying how many undulating numbers are multiples of the primes p from 2 to 71. In black the ideal line 1/p.
Undulating numbers can also be... (you may click on names or numbers and on + to get more values)
a-pointer
101
181
ABA
242
aban
101
121
131
+
989
abundant
252
272
282
+
48484848
admirable
282
464
474
+
89898
alt.fact.
101
alternating
101
121
141
+
989898989
amenable
101
121
141
+
989898989
apocalyptic
434
646
686
+
29292
arithmetic
101
131
141
+
9898989
balanced p.
373
binomial
171
252
595
+
575757
brilliant
121
323
737
+
38383
c.decagonal
101
151
c.heptagonal
1919191
c.nonagonal
595
5050
c.octagonal
121
c.pentagonal
141
181
c.square
181
313
545
+
6161
c.triangular
15151
cake
232
2626
Carol
959
Chen
101
131
181
+
1616161
congruent
101
141
151
+
9898989
constructible
272
cube
343
Cullen
161
Cunningham
101
242
323
+
232323
Curzon
141
393
414
+
161616161
cyclic
101
131
141
+
9595959
D-number
141
303
393
+
3737373
d-powerful
262
373
2626
+
43434
de Polignac
373
757
959
+
73737373
decagonal
232
2626
151515
deceptive
4141
13131313
29292929
+
101010101
deficient
101
121
131
+
9898989
dig.balanced
141
202
212
+
181818181
Duffinian
121
161
171
+
9797979
eban
4040
6060
economical
101
121
131
+
5656565
emirpimes
1313
1717
3131
+
9797
equidigital
101
121
131
+
5656565
esthetic
101
121
212
+
989898989898989
evil
101
141
202
+
989898989
fibodiv
323
646
969
Friedman
121
343
frugal
343
gapful
121
242
363
+
56565656565
Giuga
858
good prime
101
191
727
929
happy
262
313
383
+
8585858
Harshad
171
252
414
+
9090909090
heptagonal
616
4141
60606
hex
919
hexagonal
5151
hoax
202
424
454
+
25252525
Hogben
343
757
10101
Honaker
131
house
434
iban
101
121
141
+
747474
idoneal
232
inconsummate
161
272
383
+
959595
interprime
282
363
393
+
50505050
Jacobsthal
171
junction
101
202
212
+
49494949
Kaprekar
5050
7272
818181
+
85858585858585
Lehmer
595
949
4141
+
61616161
lucky
141
151
171
+
9696969
magic
505
magnanimous
101
modest
515
545
818
+
1919191919
Moran
171
1818
13131
+
73737
Motzkin
323
nonagonal
474
969
595959
nude
212
424
515
+
424242424
oban
303
313
323
+
989
odious
121
131
151
+
929292929
palindromic
101
121
131
+
989898989898989
palprime
101
131
151
+
737373737373737
pancake
121
191
232
panconsummate
121
353
pandigital
141
898
8585
+
434343
partition
101
pentagonal
1717
pernicious
121
131
151
+
9797979
power
121
343
484
+
69696
powerful
121
343
484
+
69696
practical
252
272
414
+
6969696
prim.abundant
272
282
464
+
89898
prime
101
131
151
+
91919191919
pronic
272
Proth
161
353
545
+
929
pseudoperfect
252
272
282
+
989898
repunit
121
343
585
+
101010101010101
Ruth-Aaron
949
5959
6868
19191919
self
121
323
424
+
797979797
semiprime
121
141
161
+
9797979
sliding
101
1010
Smith
121
202
454
+
90909090
Sophie Germain
131
191
sphenic
282
434
474
+
9898989
square
121
484
676
69696
star
121
181
strobogrammatic
101
181
808
+
818181818181818
strong prime
101
191
727
+
1616161
super Niven
1010
2020
3030
+
909090
super-d
131
181
454
+
9696969
tau
232
252
424
+
696969696
tetrahedral
969
triangular
171
595
5050
5151
truncatable prime
313
353
373
+
797
twin
101
151
181
+
1212121
Ulam
131
282
363
+
8282828
unprimeable
515
535
626
+
8989898
untouchable
262
292
474
+
969696
upside-down
1919
2828
3737
+
91919191919191
wasteful
171
202
212
+
9898989
weak prime
131
151
181
+
1212121
Woodall
191
323
383
Zuckerman
212
1212
13131
Zumkeller
252
272
282
+
89898