A prime number  is a Sophie Germain prime
if
is a Sophie Germain prime
if 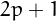 is also a prime.
is also a prime.
![\[
2C\frac{n}{(\ln n)^2} \approx 1.32\frac{n}{(\ln n)^2}
\]](https://speed.lescigales.org/xypor/index.php?q=aHR0cHM6Ly93d3cubnVtYmVyc2FwbGVudHkuY29tL3NldC9Tb3BoaWVfR2VybWFpbl9wcmltZS9waWMuNC5wbmc%3D) such primes, where
such primes, where
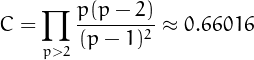 2}\frac{p(p-2)}{(p-1)^2}\approx 0.66016
\]">
(in which the product is over all odd primes
2}\frac{p(p-2)}{(p-1)^2}\approx 0.66016
\]">
(in which the product is over all odd primes  )
is the so-called Hardy–Littlewood's twin prime constant.
)
is the so-called Hardy–Littlewood's twin prime constant.
The name originates from the fact that in 1823 Sophie Germain
proved that one subcase of the Fermat Last Theorem
holds for all the prime exponents such that
is also prime.
It is conjectured that there are infinitely many Sophie Germain primes,
and that up to there are approximately
Up to there are 1822848478 Sophie Germain primes,
and the formula above underestimates the actual number by about 5.4%.
The first Sophie Germain primes are 2, 3, 5, 11, 23, 29, 41, 53, 83, 89, 113, 131, 173, 179, 191, 233, 239, 251, 281, 293, 359, 419 more terms
Pictorial representation of remainders (mod 2, 3, ...,11) frequency. For a table of values and more details click here
Sophie Germain primes can also be... (you may click on names or numbers and on + to get more values)
a-pointer
11
293
1409
+
9999166601
9999555551
aban
11
23
29
+
9999000983
10000000343
alternating
23
29
41
+
989894981
989898341
amenable
29
41
53
+
999998789
999998801
apocalyptic
251
443
1019
+
29723
29873
arithmetic
11
23
29
+
9999299
9999653
balanced p.
53
173
593
+
9999922643
9999994883
bemirp
1601
1901
10061
+
1986199601
1988809001
c.decagonal
11
281
911
+
9830843111
9892129601
c.heptagonal
953
5741
10781
+
9929501513
9956364461
c.square
41
113
761
+
9972250313
9997687013
Chen
11
23
29
+
99999551
99999611
congruent
23
29
41
+
9998903
9999653
Cunningham
1601
12101
25601
+
9894280901
9960040001
Curzon
29
41
53
+
199999661
199999853
cyclic
11
23
29
+
9999299
9999653
d-powerful
89
2063
2753
+
9972569
9978833
de Polignac
251
509
809
+
99997619
99998999
deficient
11
23
29
+
9999299
9999653
dig.balanced
11
41
653
+
199987241
199993361
economical
11
23
29
+
19999253
19999379
emirp
113
179
359
+
199998923
199999661
equidigital
11
23
29
+
19999253
19999379
esthetic
23
89
12101
+
6765654323
6787654343
Eulerian
11
1013
evil
23
29
53
+
999998789
999998801
fibodiv
911
1499
8612801
916666661
Fibonacci
89
233
Friedman
12101
15629
28559
+
946733
953321
Gilda
29
683
good prime
11
29
41
+
199882961
199883693
happy
23
239
293
+
9998903
9999299
Honaker
131
1049
2141
+
999412313
999821261
hungry
2003
iban
11
23
41
+
777041
777173
inconsummate
173
431
443
+
998969
999623
Jacobsthal
11
683
43691
2796203
junction
113
509
719
+
99996353
99996749
katadrome
41
53
83
+
764321
986543
Kynea
23
Leyland
593
lonely
23
53
24281
38501
10938023
Lucas
11
29
3010349
54018521
m-pointer
23
1111361
3221111
+
2232113111
4111311131
magnanimous
11
23
29
+
800483
48804809
metadrome
23
29
89
+
134789
145679
modest
23
29
89
+
1994010989
1997009009
nialpdrome
11
41
53
+
9999977441
9999988811
nude
11
oban
11
23
29
+
911
953
odious
11
41
131
+
999998903
999999191
Ormiston
1931
34631
37379
+
1999987331
1999995713
palindromic
11
131
191
+
997909799
999212999
palprime
11
131
191
+
997909799
999212999
pancake
11
29
191
+
9948327041
9991960931
panconsummate
11
23
53
89
239
pandigital
11
partition
11
pernicious
11
41
131
+
9998903
9999299
Perrin
29
43721
plaindrome
11
23
29
+
6888899999
7777778999
prime
11
23
29
+
9999999701
10000000343
primeval
113
1013
1003679
100234679
Proth
41
113
641
+
9975365633
9996206081
repdigit
11
repunit
28792661
78914411
943280801
7294932341
self
53
233
659
+
999990581
999998903
self-describing
10311533
12313319
15333119
+
4419414113
4441411913
sliding
11
29
641
strobogrammatic
11
16091
1881881
+
6986889869
6988968869
strong prime
11
29
41
+
99998603
99999611
super-d
131
281
419
+
9998633
9998903
tetranacci
29
trimorphic
251
74218751
truncatable prime
23
29
53
+
9391564373
9439663853
twin
11
29
41
+
999998141
999999191
uban
11
23
29
+
9093000029
9096000029
Ulam
11
53
131
+
9986849
9991259
undulating
131
191
upside-down
1289
1559
7193
+
9992648111
9993557111
weak prime
23
83
89
+
99999551
99999563
weakly prime
3326489
5575259
7469789
+
9997470629
9999130709
Woodall
23
191
4373
590489
Zuckerman
11
zygodrome
11
44111
77711
+
9996661133
9999988811